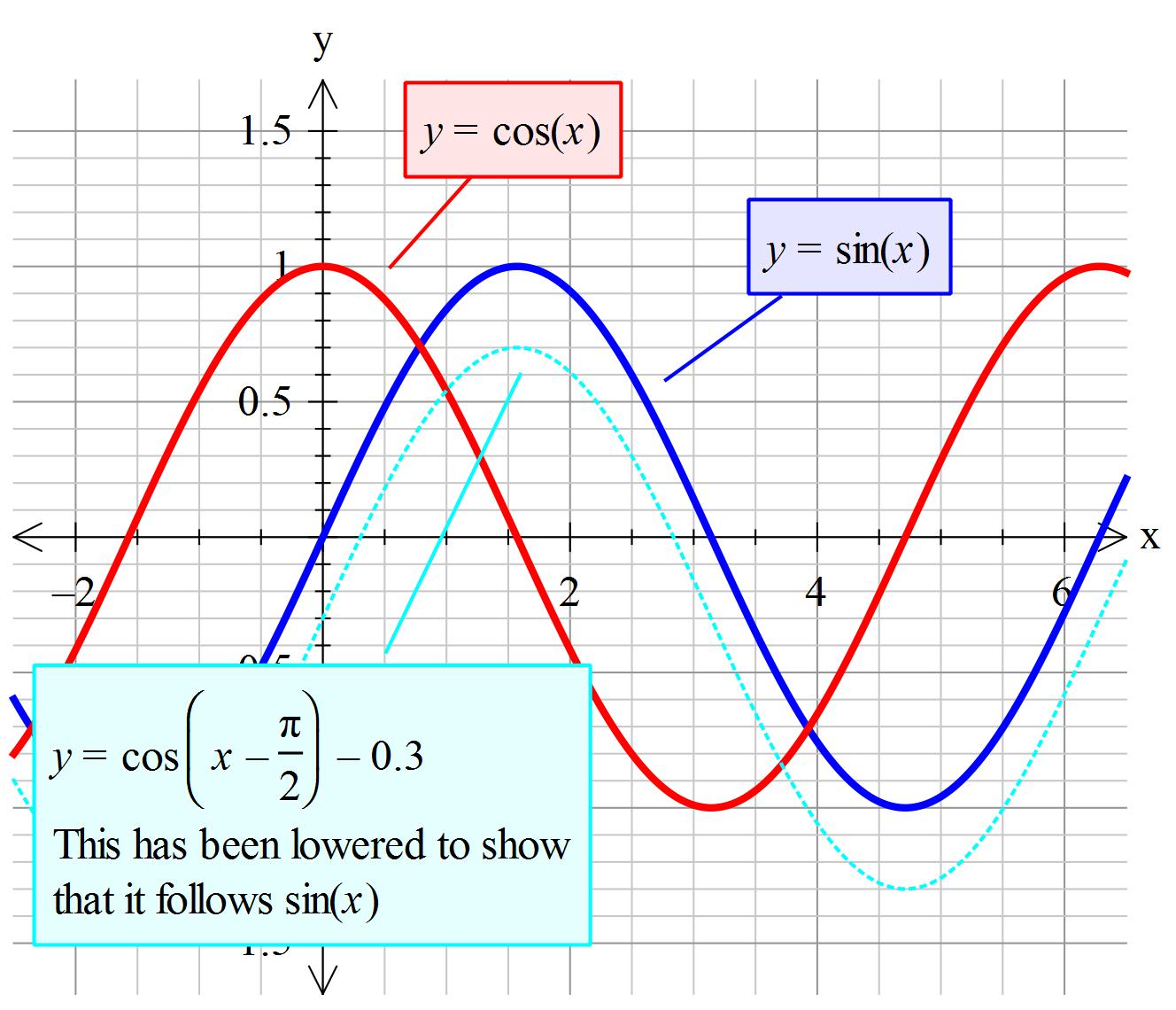
y=sinθ1とy=sin(θπ/4)のグラフの書き方を教えてください。 数学 解決済 教えて!goo⋆ 逆三角関数のグラフ y =sinx y =Arcsinx 2 1 2 1 21 21x y O y =Arcsinx 1 212x y O y =cosx y =Arccosx 1 2 ˇ 1 ˇ 211x y O y =Arccosx 1 ˇ 21x y O y =tanx y =Arctanx22 2しかし、グラフから分かるように cosθ=sin(θ+π/2) であるから、 y=cosθ のグラフは、 y=sinθ のグラフを θ の方向に -π/2 だけ(負の方向に π/2 だけ)平行移動したものである。
How Do You Find The Equation Of The Line Tangent To The Graph Of Y Sin X At The Point X Pi Socratic
Y=sin(θ+π/2) グラフ
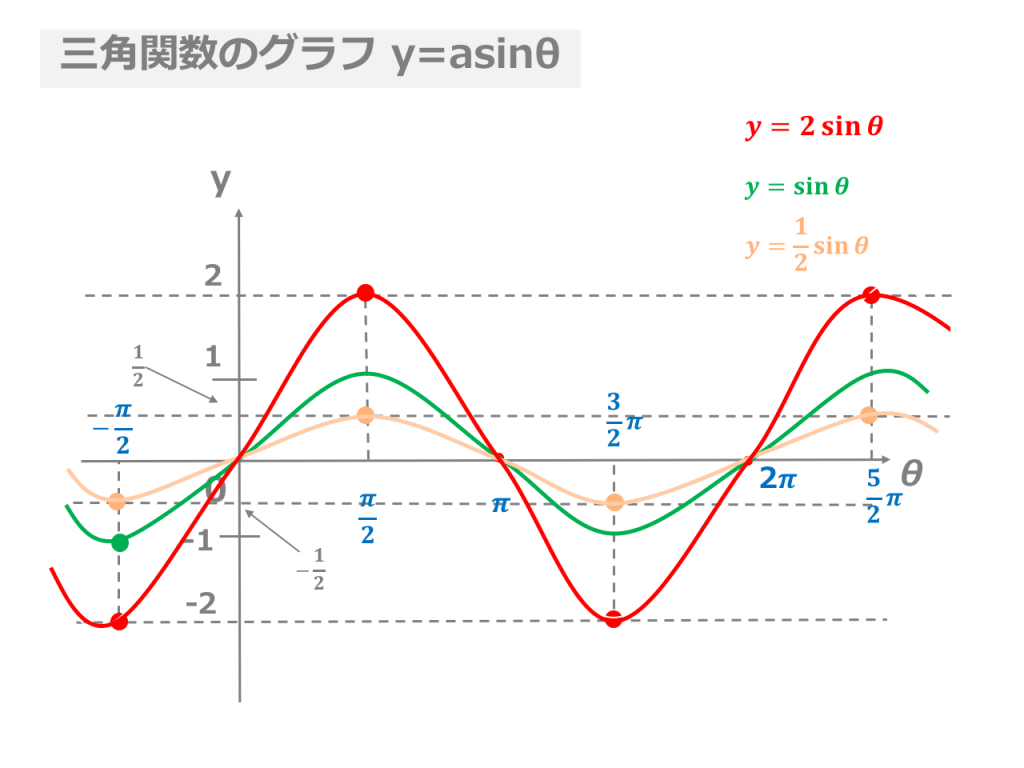
Y=sin(θ+π/2) グラフ-θ d θ の積分を図形を用いて直感的に理解する. 左側の図は 単位円 ,右側の図は y =sinθ y = sin θ のグラフである. 図において赤色の面積と青色の面積は等しい. ∫ π 2 0 sinθdθ =−cosθπ 2 0 = −cos π 2 cos0 = 1 ∫ 0 π 2 sin θ d θ = − cos Y = sinθ のグラフは,y = sinθ のグラフを y軸方向に 倍 したグラフです。 → y = 2 sinθ のグラフは,Step1の y = sinθ のグラフを y軸方向に 2 倍 します。 ≪Step3 y = sin(θ ) のグラフをかく≫ y = sin(θ ) のグラフは,Step2の y = sinθ のグラフを θ軸方向に だけ平行移動 します。




7 のtanの式とグラフについてです Clear
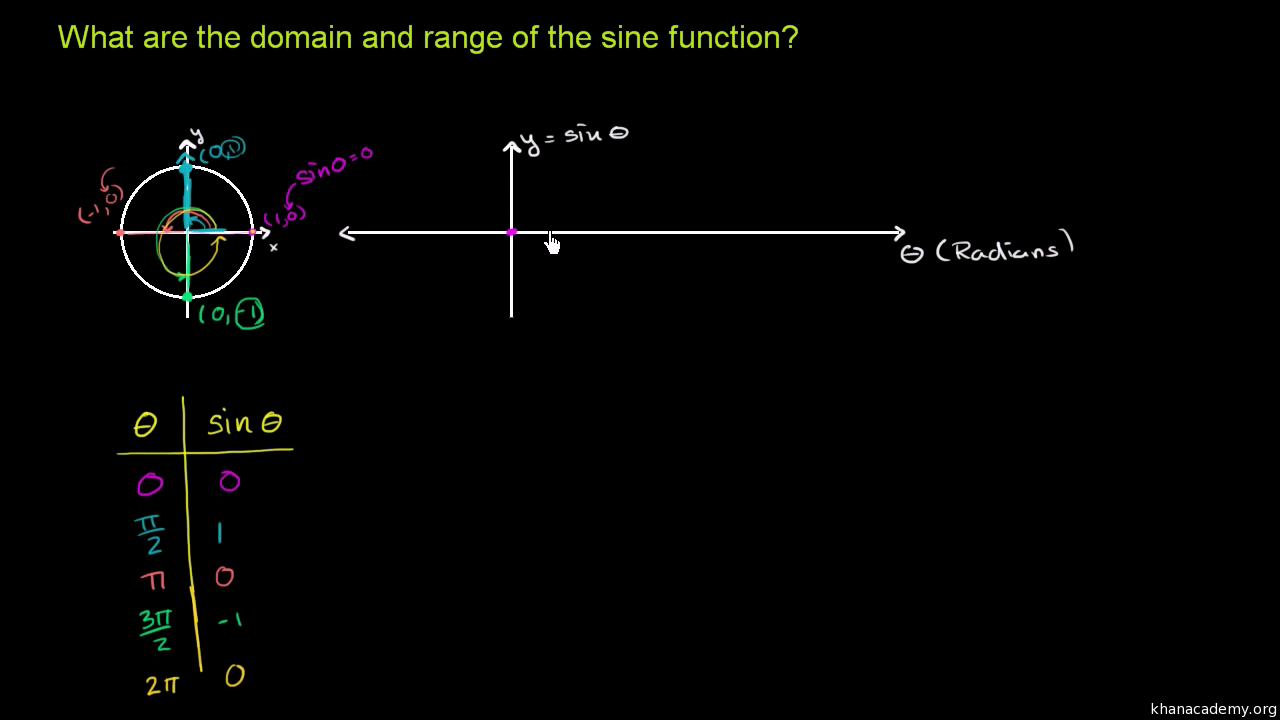
∫ π 2 0 e¡Rsinθdθ sinθ ≥ 2θ π (0 ≤ θ ≤ π 2) であるから(図4) O y θ y = 2θ π y = sinθ 1 π 2 π 2π 3π sinx x のグラフ 6/8Let a line through the origin intersect the unit circle, making an angle of θ with the positive half of the xaxisThe x and ycoordinates of this point of intersection are equal to cos(θ) and sin(θ), respectivelyThis definition is consistent with the rightangled triangle definition of sine and cosine when 0° < θ < 90° because the length of the hypotenuse of the unit circle is always π/2<θ≦π/2の範囲で、関数y=sinθは単調に増加しますか? 数学 解決済 教えて!goo
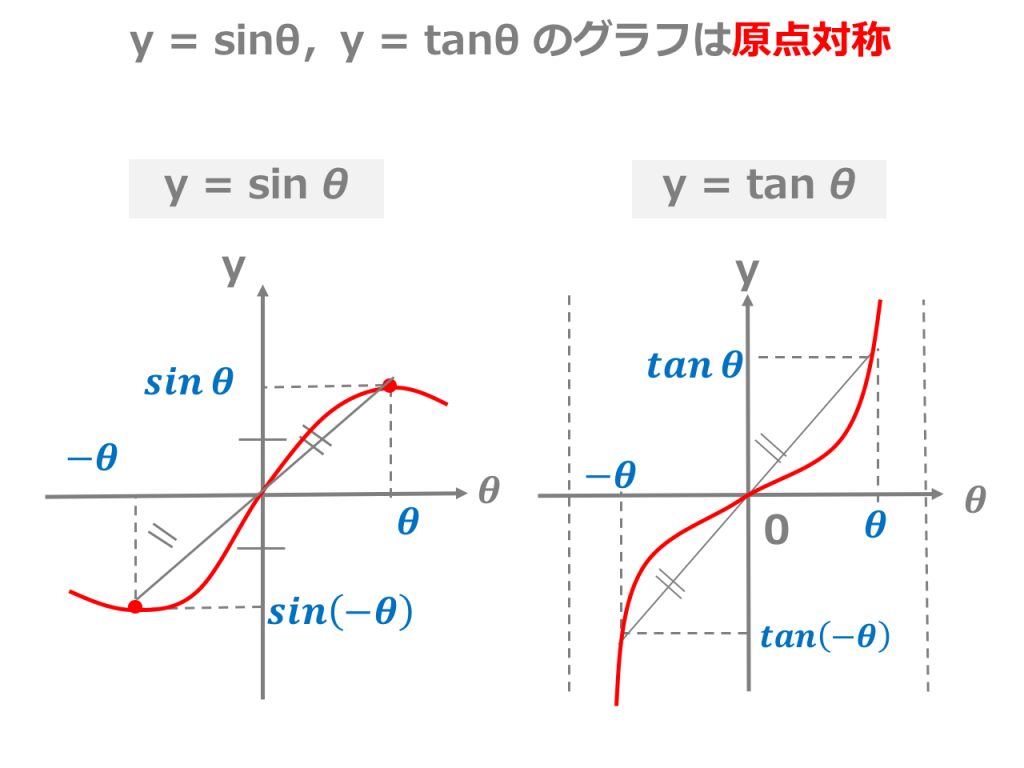
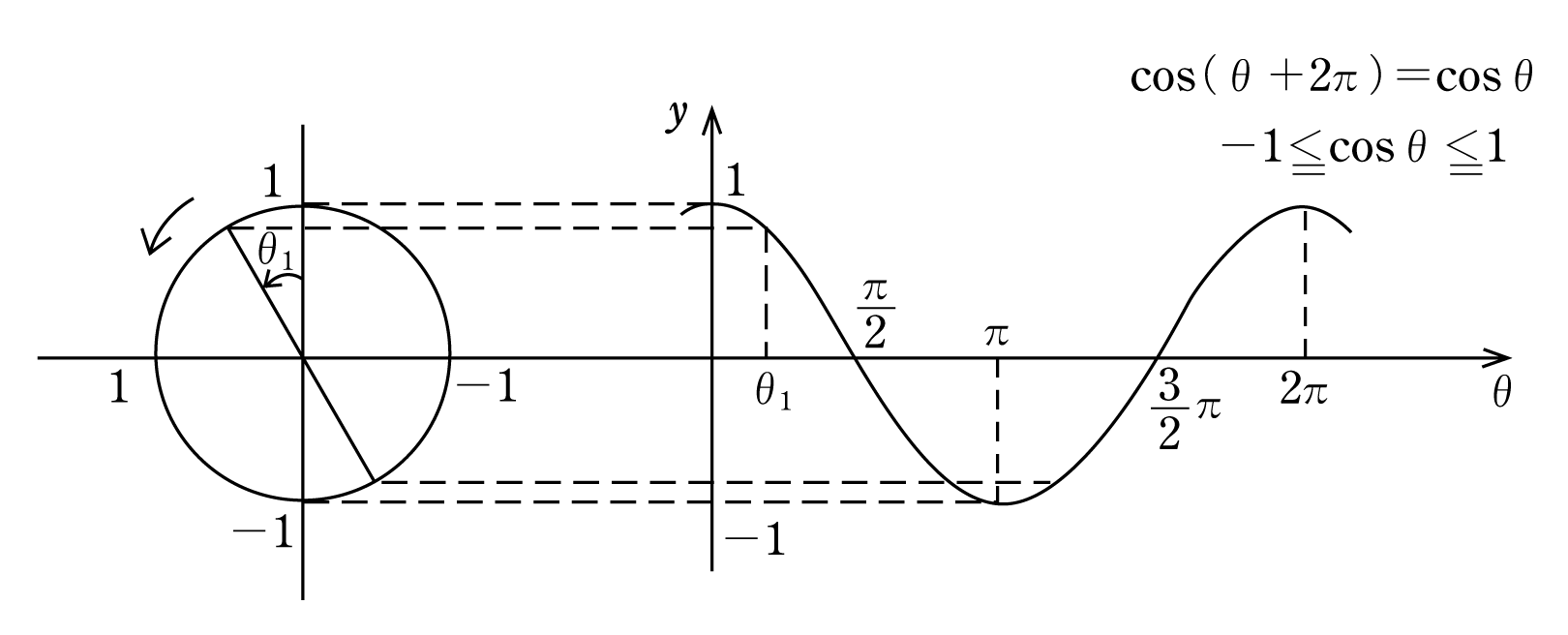
0 votes 1 answer If a directrix of a hyperbola centred at the origin and passing through the point (4, –2√3 ) is 5x = 4√5 and its eccentricity is e, then For some θ ∈ (0,π/2) , if the eccentricity of the hyperbola, x^2–y^2sec^2θ = 10 is asked in Mathematics by Susmita01 (462k points) jee main ;( sin(-θ)=-sinθ ) グラフは左右に無限までのびた沿である。 左に π/2 だけ平行移動すると y=cosθ のグラフとなる。 2° y=sin(θ+π/2)=cosθ y=cosθ 3°y=cosθ ・・・ ②
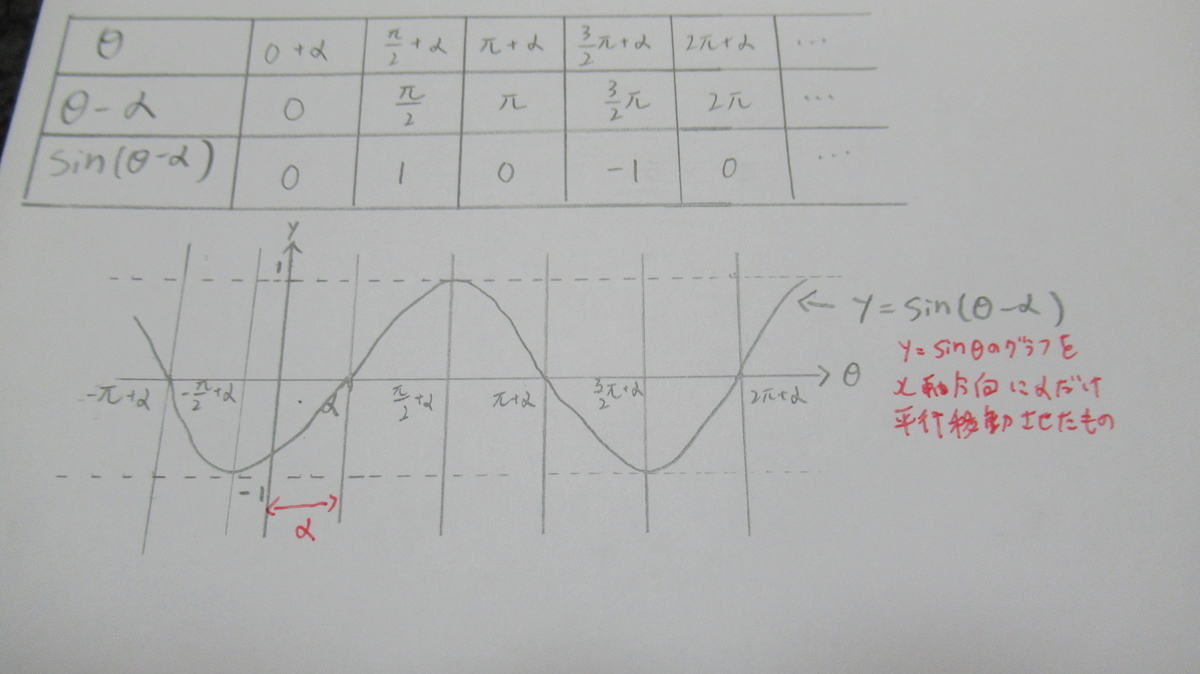
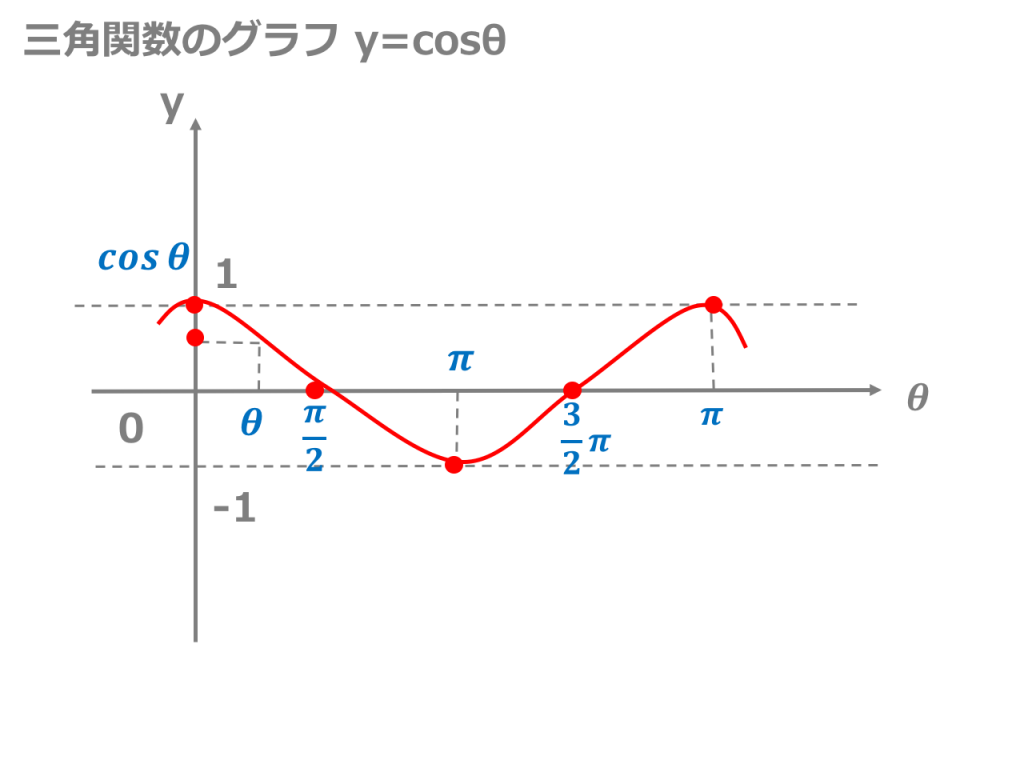
代表的なx,yの数値を確認することで、y=sin^2xのグラフがどうなるのかについて確認します。 まずは、 ・x=0の場合はsinx自体が0のため、y=sin^2x=0となります。 ・x=30度の場合はsin30度が1/2のため、y=sin^2x=1/4となります。 ・x=45度の場合はsin30度が√2/2のため、y=sin^2x=1/2となります。(2) cos θ のグラフ:取りうる値は-1 ≦ cos θ ≦1 cos( θ 2 π) = cos θ だから、sin と同様2πごとに同じ値を取る周期関数 sin( θ ) = cos θ だからsin のグラフを だけシフトしたグラフ@ y= sin (x−) ̂Ƃ ɁC x ̐ ̌ ړ ܂ D @ y= sin (x ) ̂Ƃ ́C x ̌ − ړ ܂ D



3分でわかる 三角関数のグラフの描き方 合格サプリ



Graph Of Y Sin X Video Trigonometry Khan Academy
46 三角形から円運動へ 47 第 2章 一般の三角関数 O 05 0 5 0 5 sinθ θ θ cos P y x -1 -10 0 1 10 一般角 円運動とサイン、コサインを考えていくと、動径と x 軸の正の方向 とのなす角は360°より大きくてもよいし、マイナスでもよいことになっ グラフとPythonを使って身近なものに触れたかった。 実際にやってみる 数学Ⅱ・数学B 大1問〔1〕(1)問題A 問題 関数 y = sin θ√3 cos(θ) (0 y=√x, y=√1x, y=sin(x), y=sin(π/2x)のグラフの考え方がいまひとつわかりません。 例えば、あえて逆関数を考えずに、y=√1xをy=√xをx軸方向に1だけ平行移動したものと 考えると解答と違ってしまいます。 / 解答がどのようなものかわからないので、正確なこ



振動と波動の準備 三角関数の復習 定義と微分 三角関数は 物理でよく使います Ppt Download




解説読んでも分かりません 教えてください Clear
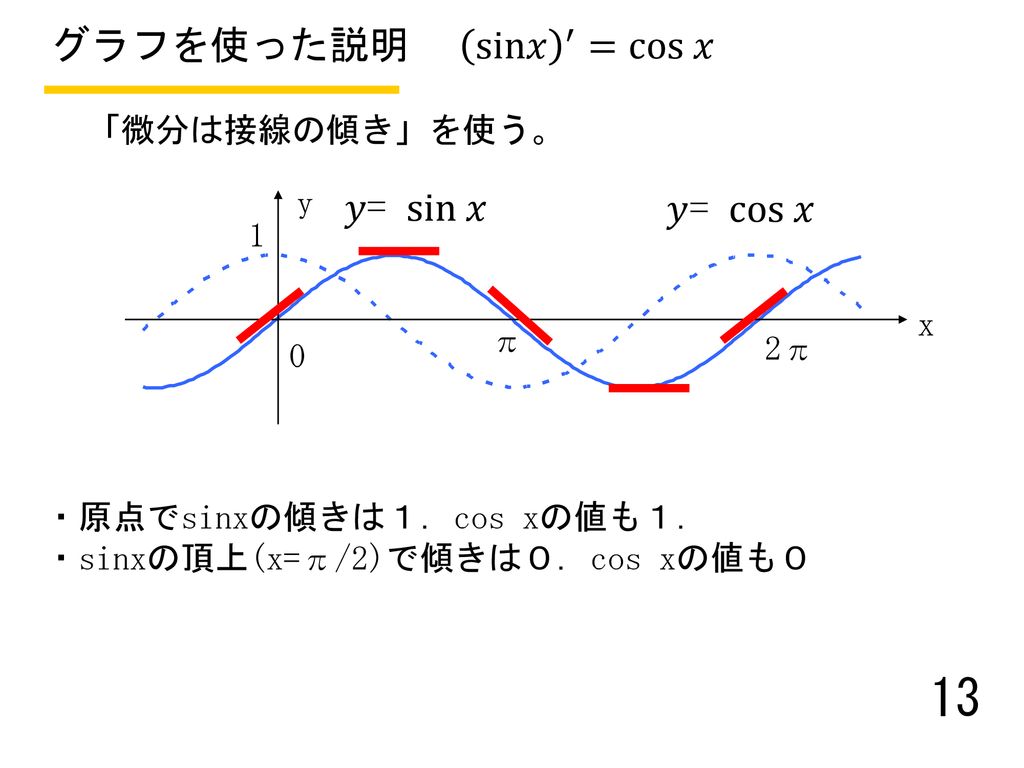
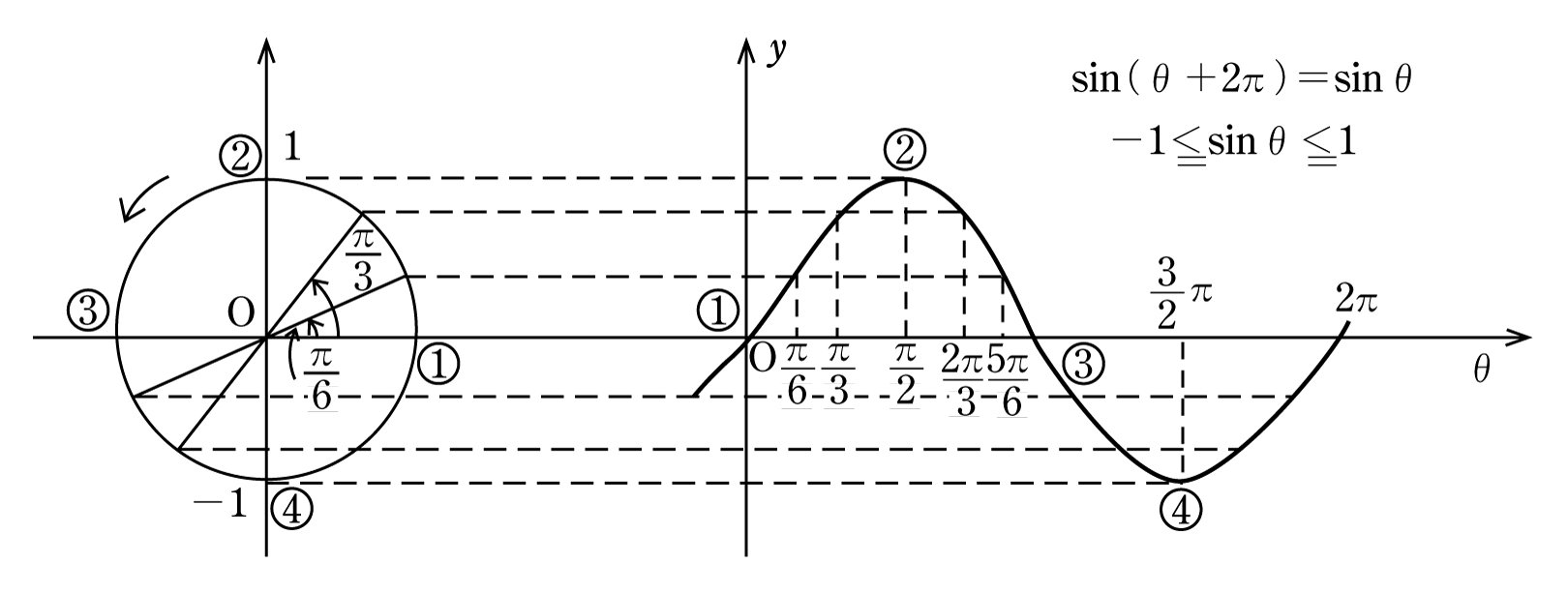
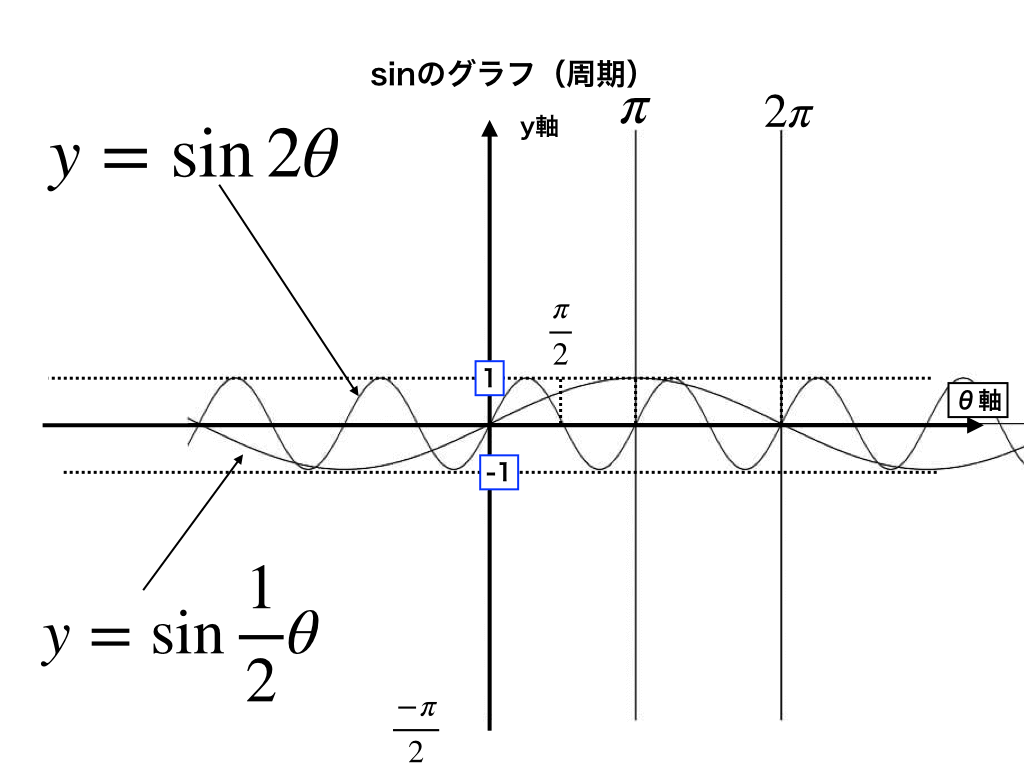
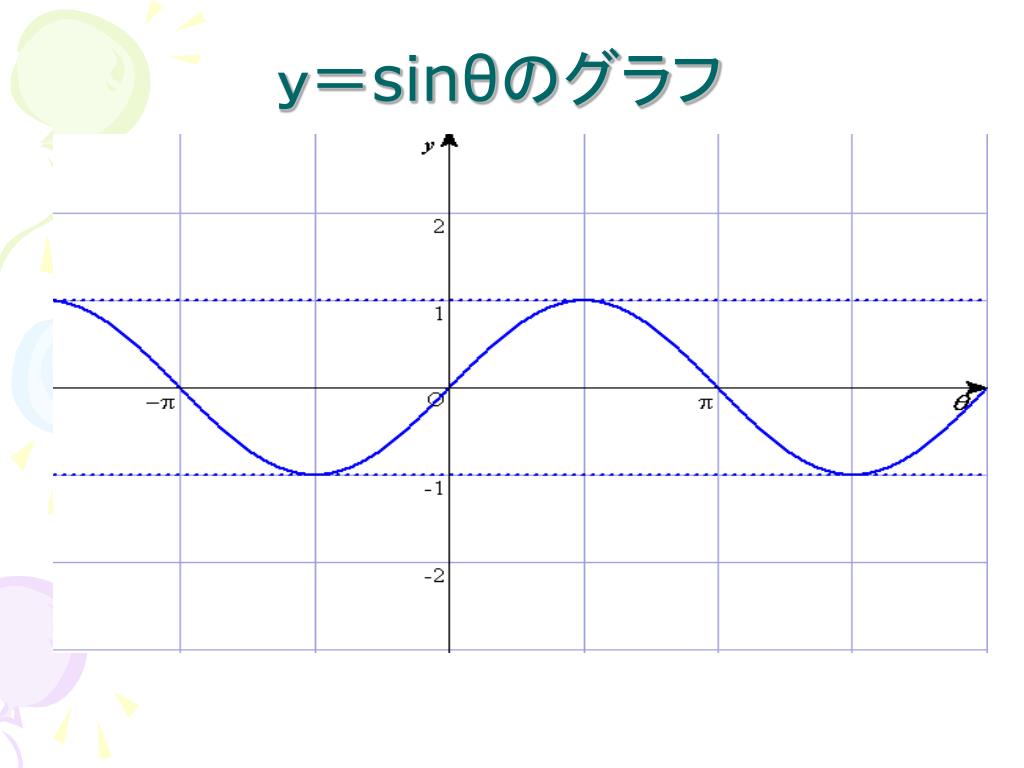
Prof Dr Aris Christidis • WS 18 / 19 Globale Operationen Hough Grundidee Suche nach gemeinsamer Steigung m und Achsenabschnitt b, die Punkte mit bekannten KoordinatenY= sin θ のグラフを描くには、右のような対応表(θの値と y の値を表にしたもの)を作り、求めた座標(θ , y )を結んでいく。 この y= sin θ のグラフは、以下の解説を通じて何度も登場する基本の形なので、しっかりとイメージに刻んでおくことが重要。 特に、次の点は確実に言えなくてはならない。 (1) sin 0 ° =0 だから θ =0 ° のとき y=0 (2) sin 90 ° =1 だY=sin(2θ)のグラフ(y=sinθのグラフとどこがどう違うのだろう?) 1 周期が1/2になっている。周期とは動点Pが出発点に初めてもどるまでのθ(横軸の長さ)。 y=sinθの周期は2π、y=sin(2θ)の周期はπ。 2 振動数が2倍になっている。
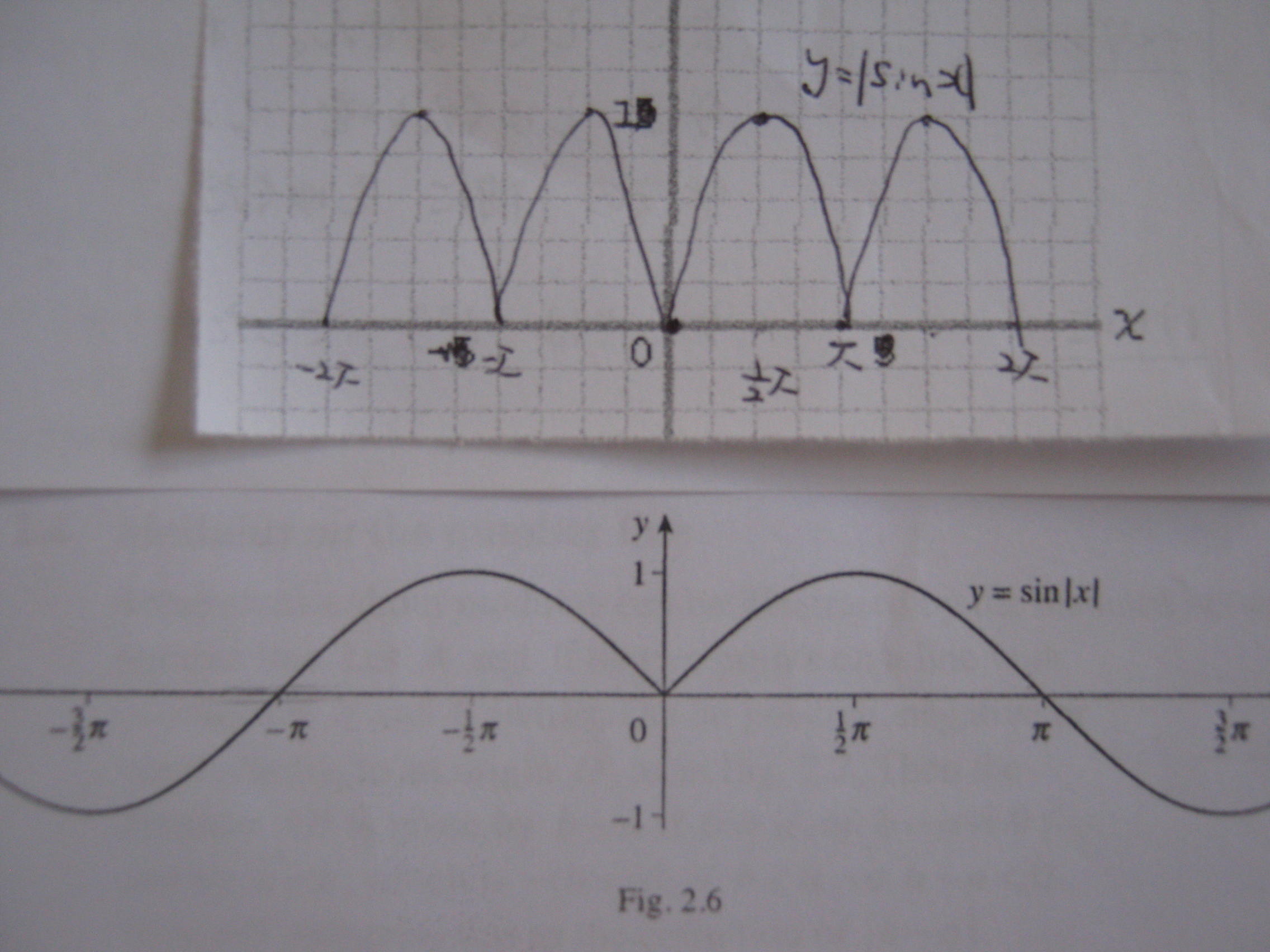



グラフの答え合わせと説明お願い出来ますか 絶対値 Okwave



Graph Y 1 3 Cos X P 2 Youtube
はsinc関数と呼ばれる有名な関数である。 y = sin x x y=\dfrac {\sin x} {x} y = xsinx という関数に関連する話題を整理しました。 目次 sinx/xの極限が1になることの証明 sinx/xの拡張 sinx/xのグラフSolution Given x = a (1 cos θ) dx/dθ = a sin θ y = a (θ sin θ) dy/dθ = a acos θ dy/dx = (a a cos θ)/a sin θ = (1 cos θ)/sin θ = 2 (cos 2 θ/2)/ 2 sin θ/2 cos θ/2 = – cot θ/2グラフ作成専用Webアプリ(関数グラフ、方程式の探究、データのプロット、スライダー利用、等々) 関数グラフ Calculator Suite




In The Graph Of The Function Y Sin 8 As 8 C Gauthmath



Http College Cengage Com Mathematics Stewart Student Data Ch11 Pdf
If x = cos θ and y = sin^3 θ, then prove that yd^2y/dx^2 (dy/dx)^2 = 3sin^2 θ(5cos^2 θ 1), asked in Mathematics by Samantha ( 3k points) continuity and differntiability三角関数のグラフをかくときには, y =sin θ , y =cos θ , y =tan θ のグラフをもとにしてかくとよいでしょう。 このとき,移動のしかたがわからなくなったら,ポイントになる点 をとって,その移動の様子を参考に考えてみてくださいね。 それでは三角関数(Trigonometric Function) 1 ピタゴラスの定理 直角三角形(right triangle) は,測量の基本と言える.直角三角形でない三角形も存在するが,どん な三角形でも補助線を設けることで,二つの直角三角形に分割することが出来る.ここが重要なポイ




46 Use The Graph Above To Find The Value Of Y Sin Gauthmath



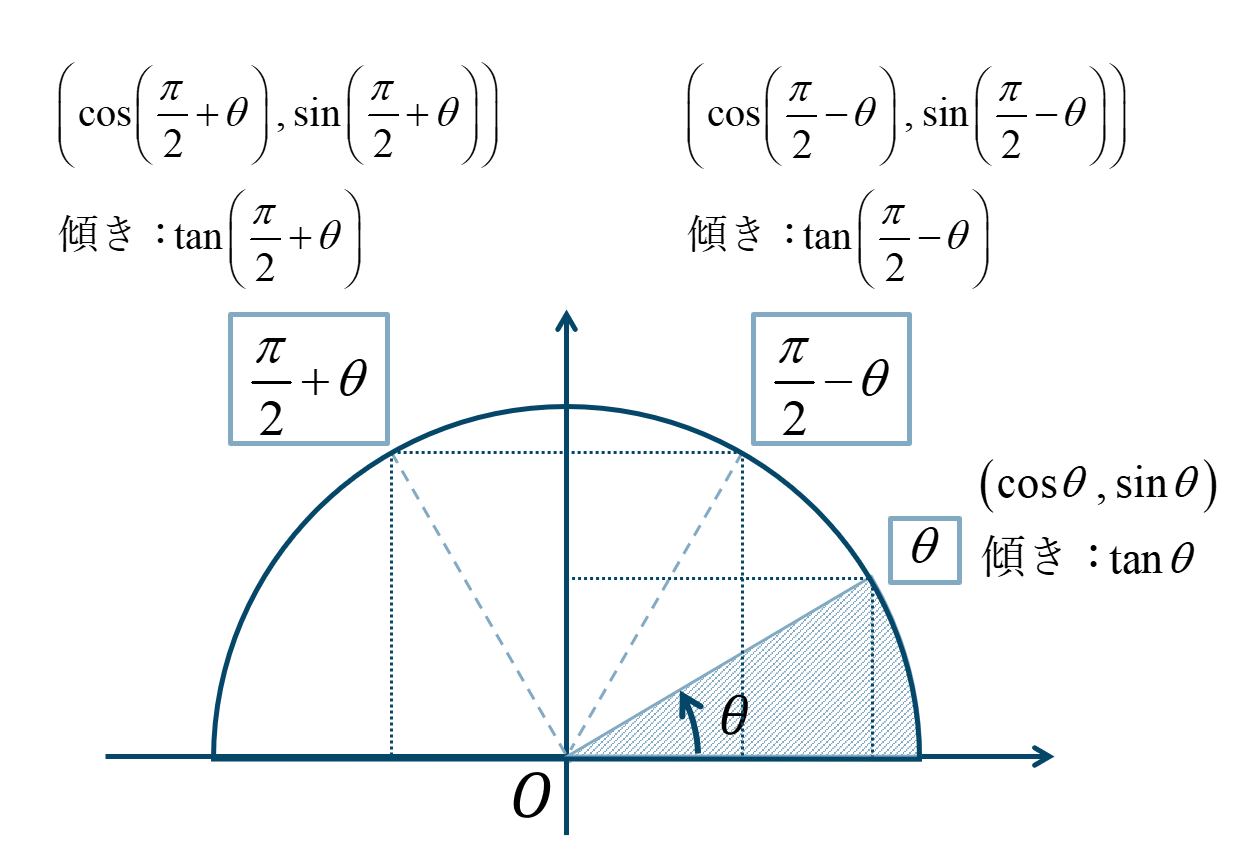
How Do You Prove Cos X Pi 2 Sin X Socratic
Try IT(トライイット)のθ と θ+(π/2)の関係の映像授業ページです。Try IT(トライイット)は、実力派講師陣による永久0円の映像授業サービスです。更に、スマホを振る(トライイットする)ことにより「わからない」をなくすことが出来ます。全く新しい形の映像授業で日々の勉強のCos(0) = 1 , cos(π/3) = 1/2 , cos(π/2) = 0 のような値をグラフにすると上記のようなグラフになります。 やっぱり2πで一周期です。 こっちは、3πまでグラフを書いてみました。List of trigonometric identities In mathematics, trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined Geometrically, these are identities involving certain functions of



三角関数のグラフの書き方を徹底解説 周期や平行移動の問題も 受験辞典



Pplato Flap Math 1 6 Trigonometric Functions
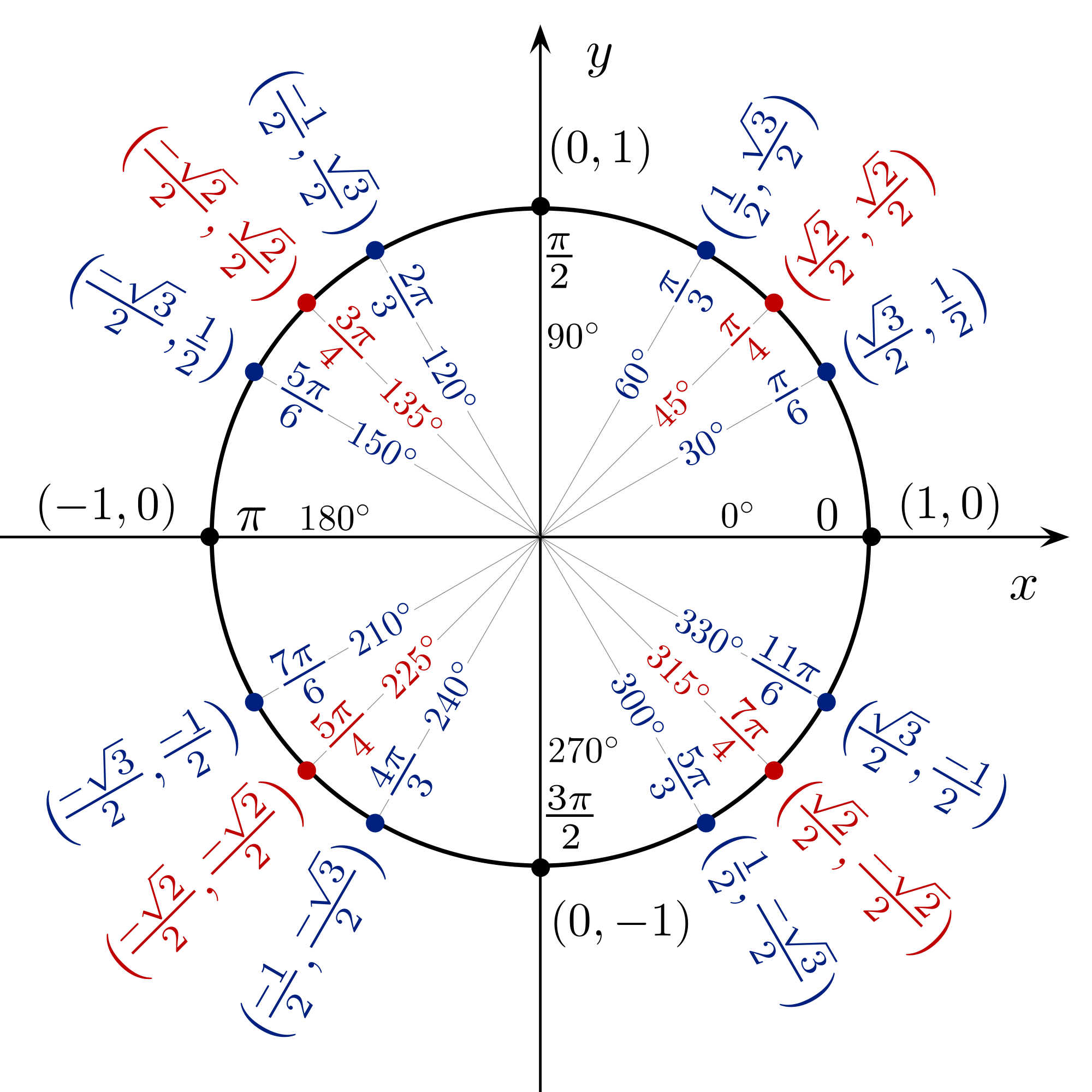
三角関数 グラフ 周期 y=sinθは2πですよね。 y=sin(θπ/2)をθ軸方向にπ/2だけ平行移動しても、2πのままなのは何故ですか 三角関数のグラフ:y=sinθのグラフ まずは、y=sinθのグラフから確認しましょう。 下の図のようになります。 三角関数のグラフ y=sinθ まずはグラフの見方を説明します。 このグラフはy軸とθ軸のグラフです。 x軸ではないので注意しましょう。 θの値が変動するにつれてどういう動きを取るかがグラフに図示されています。 などがグラフに描かれている3 θ = Arccosxとするとx = cosθ (0 ≤ θ ≤ π) このとき、− π 2 ≤ π 2 −θ ≤ π 2 であって、 sin π 2 − θ = sin π 2 cosθ− cos π 2 sinθ = cosθ = x なので、 π 2 −θ = Arcsinxと表せる。よって、 π 2 − Arccosx = Arcsinx すなわちArccosxArcsinx = π 2 O O π/2−θ π/2−θ θ θ x x x x



Question Ac026 Socratic



交流瞬時値の三角関数表示式 音声付き電気技術解説講座 公益社団法人 日本電気技術者協会
y=sinのグラフと一致します。 こちらも、y=cosθの0〜π/2の範囲を頭に思い浮かべます。 (たくさんイメージするのは大変なので) 左にπ/2移動させると、原点に点がきます。 これはy=sinθのグラフの特徴の1つです。 さらに、π〜0の範囲に、上向きの山がくるので、y = sinθということが分かります。 tan(θπ/2)数II 三角関数のグラフ y=sinθ/2 のグラフは y=sinθのグラフをθ軸方向に2倍に拡大しますがなぜ 1/2倍じゃないんですか?Y=sinθのグラフは原点に対して対称です。 (3) 奇関数 sin(θ)=sinθ より,y=sinθは奇関数であることがわかります。 π/2とπ/2など,θの符号が反対になっている所ではsinθの値も反対になっていることが表やグラフで確認できます。 (4) 周期



How Do You Find The Equation Of The Line Tangent To The Graph Of Y Sin X At The Point X Pi Socratic
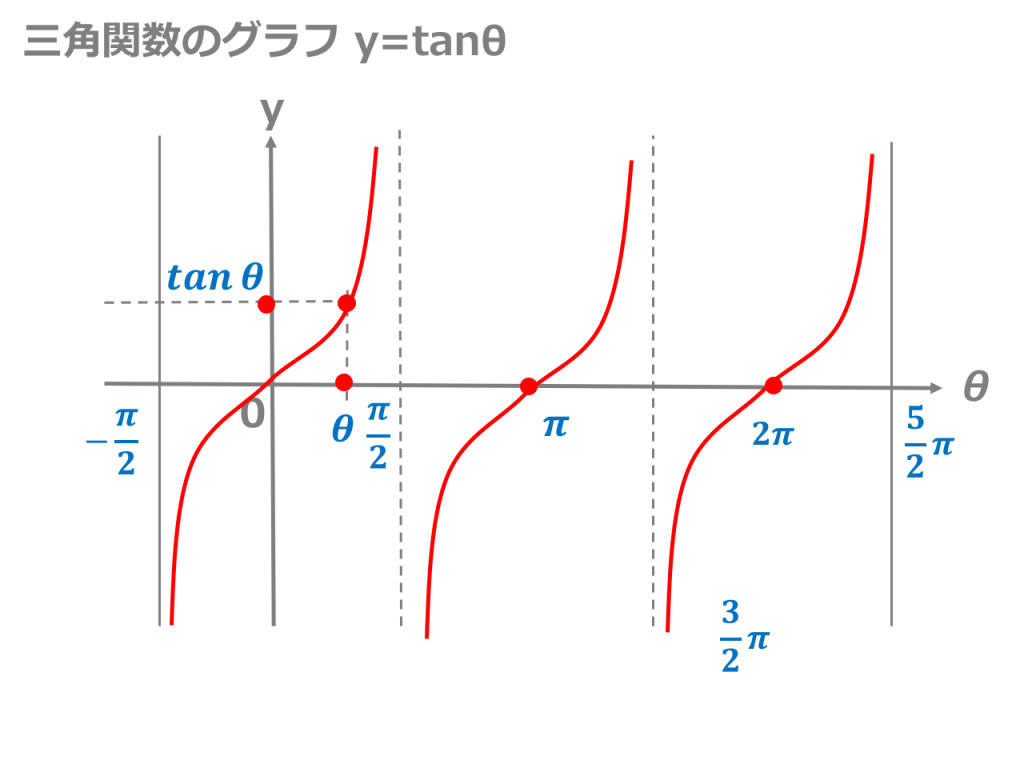



3分でわかる 三角関数のグラフの描き方 合格サプリ
= −θ のグラフの書き方について,以下の空欄にあてはまる説明を考えて埋めよ. のグラフは,y=sinθのグラフを したものである. − 4 π 4 π 2 π 4 3π π 4 5π 2 3π 4 7π 2π 4 9π θ −3 −2 −1 1 2 3 y O − 4 π 4 π 2 π 4 3π π 4 5π 2 3π 4 7π 2π 4 9π θ −3 −2 −1 1 2 3 y O − 4 π 4 π 2 π 4 3π π 4 5π 2 3π 4 7π 2π 4 9π θ −3 −2 −1 1 2 3 ySinのグラフは、 角度0から始まり、角度2π で一区切りになる波形ですよね。 今回、求めるグラフの角度は 2θ です。 始まりと終わりを求めましょう。 グラフの 始まり は、 2θ=0 より θ=0 グラフの 終わり は、 2θ=2π より θ=π となります。 これでy=sin2θの基本波形の始まりと終わり、つまり 周期がθ=π とわかりましたね。Angle, θ y = sin θ y = cos θ 0 π _ 6 1 _ 2 = 050 √ __ 3 _ 2 ≈ 087 π _ 4 π_ 3 π _ 2 b) Extend the table to include multiples of the special angles in the other three quadrants Investigate the Sine and Cosine Functions Materials • grid paper • ruler The Bay of Fundy, between New Brunswick and Nova Scotia, has the highest tides




Trig Graphs And Equations Ppt Download



Draw The Graph Of Y Sin 1 Sin X
左側の図は単位円,右側の図は y = sin θ のグラフである. 図において赤色の面積と青色の面積は等しい. ∫ 0 π 2 sin θ d θ = − cos θ 0 π 2 = − cos π 2 cos 0 = 1θ 0 pi 4 pi 2 r = sin 2θ 0 1 0 Note that sin2 θ> 0for




Grahing Y Sin X Pi 2 Youtube



Draw The Graph Of Y Sin 1 2x 1 X 2
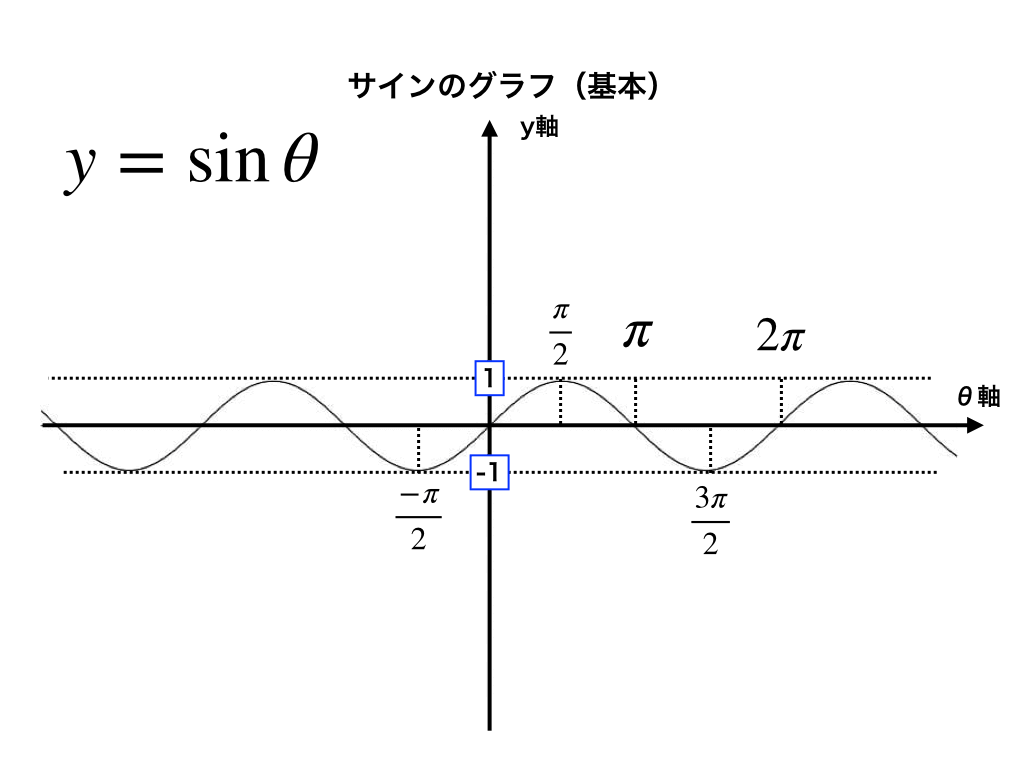



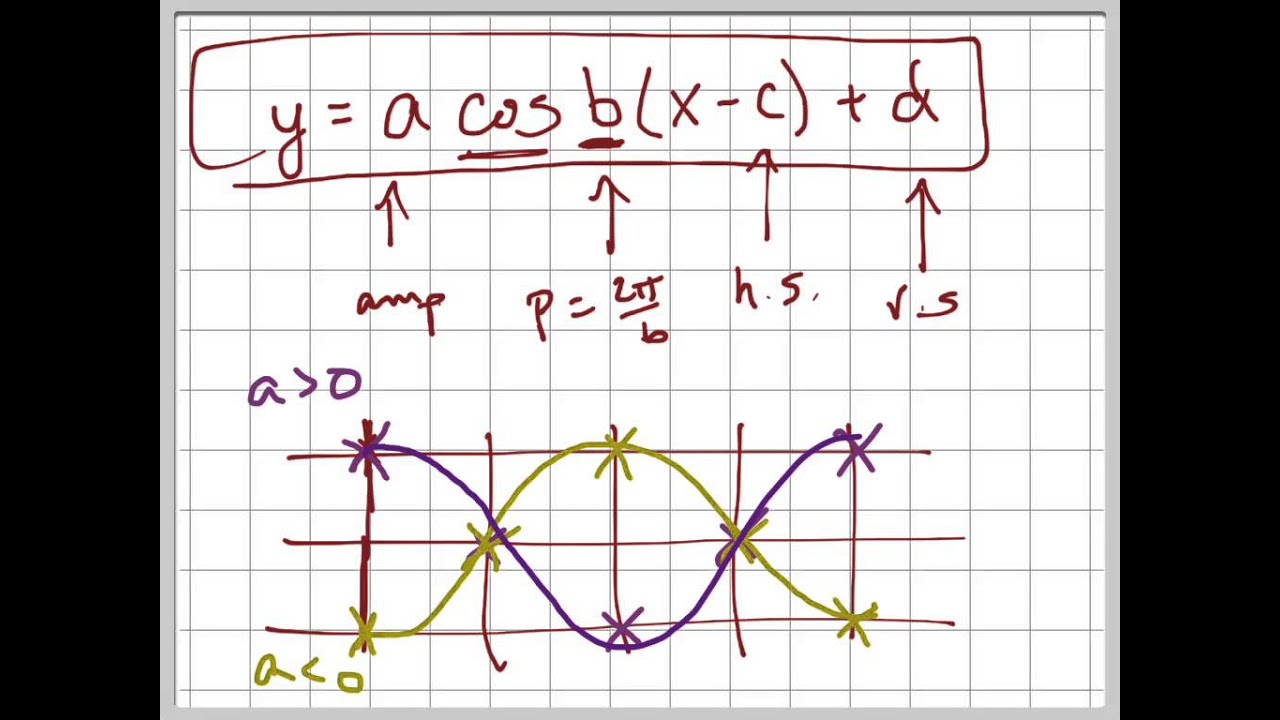
三角関数のグラフの描き方と式の平行移動 振幅 周期の読み取りを解説




Find The Area Enclosed By Circles R 3 Cos Theta And R 3 Sin Theta Mathematics Stack Exchange




Trig Graphs And Equations Ppt Download



How Do You Graph Y 1 2 Sin X Pi Socratic



3




ピンク波線部の8 P 6のとき とはどういうことでしょうか 詳しく教えてください Clear



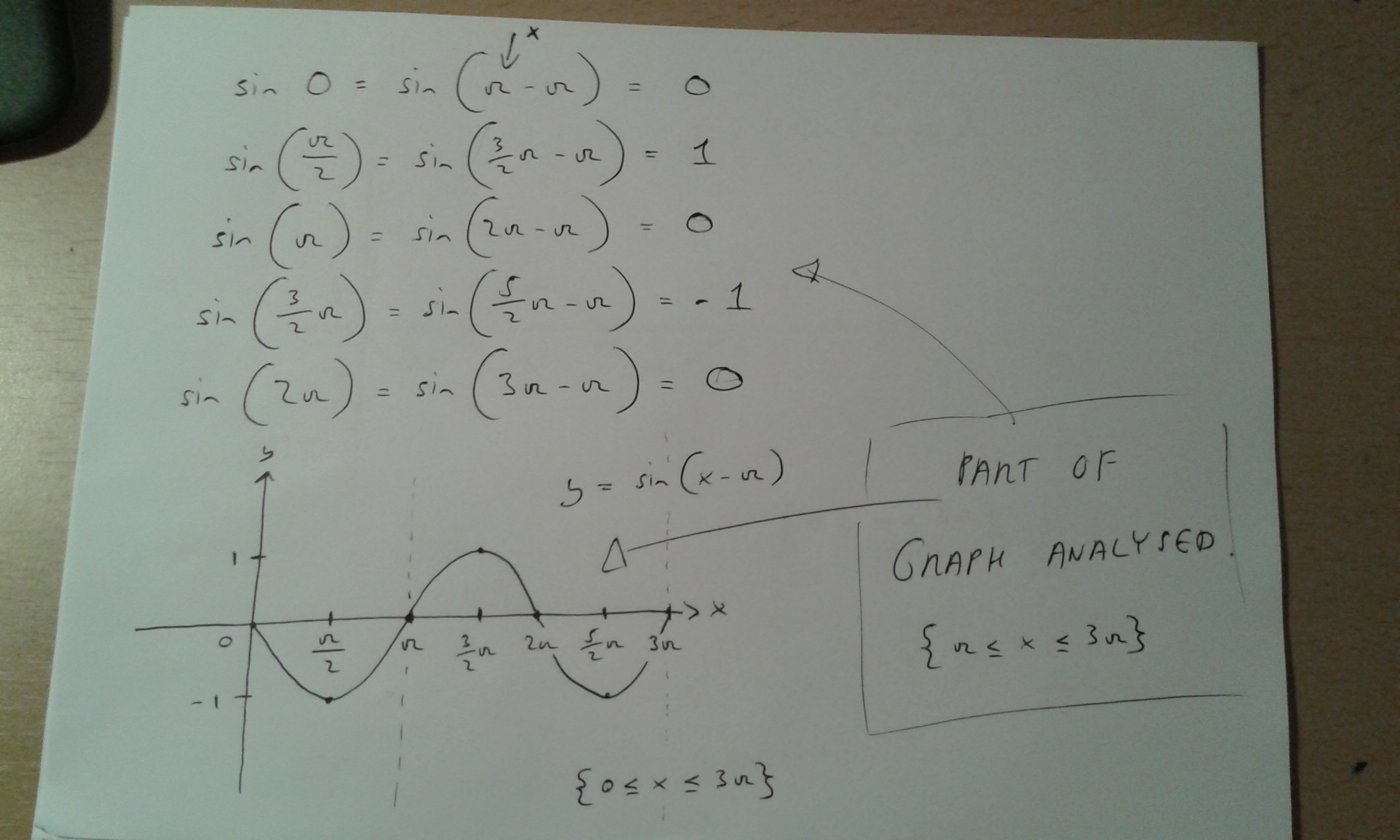
三角関数のグラフの書き方を徹底解説 周期や平行移動の問題も 受験辞典



数学 P 2 8 P 2 8の三角関数の求め方とコツ ページ 2 教科書より詳しい高校数学




Trigonometry Addition Formula For Rcos X A And R X A Ppt Download



三角関数のグラフの描き方と式の平行移動 振幅 周期の読み取りを解説



Pplato Flap Math 1 6 Trigonometric Functions
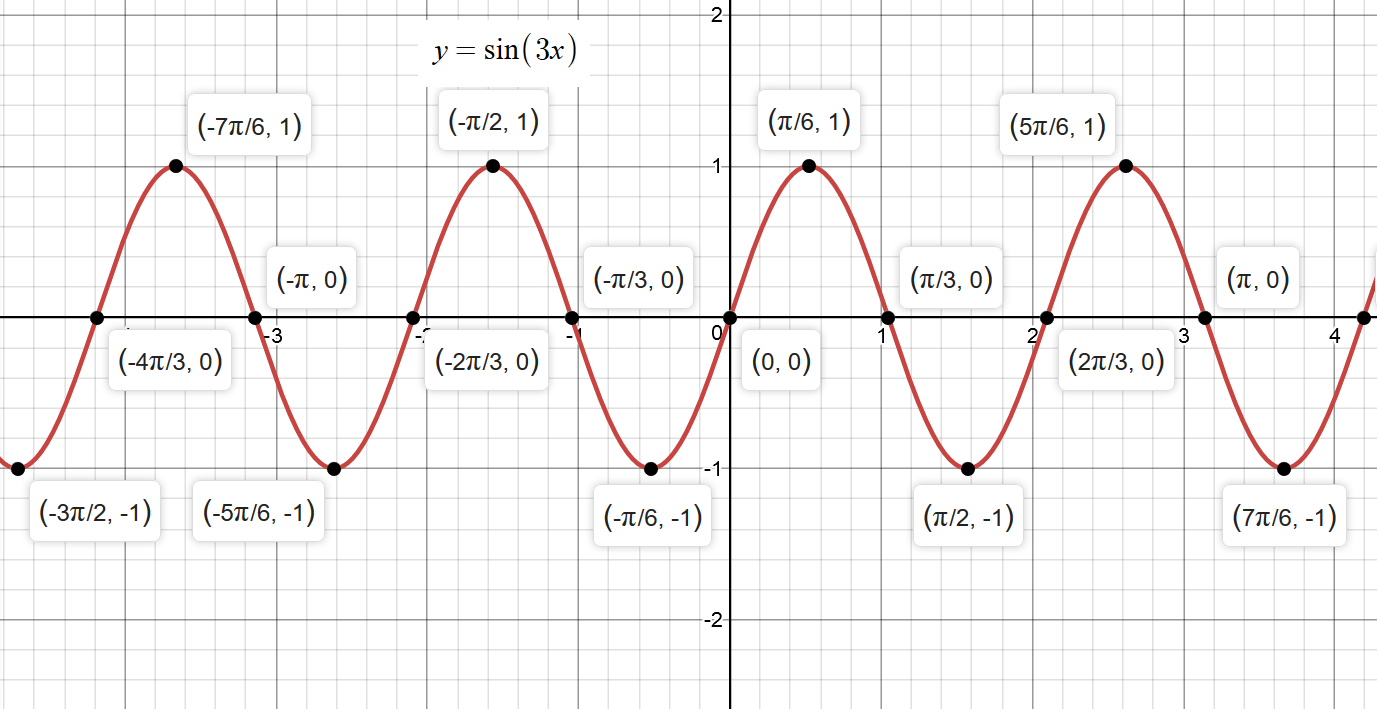



How Do You Graph Y Sin3x Socratic
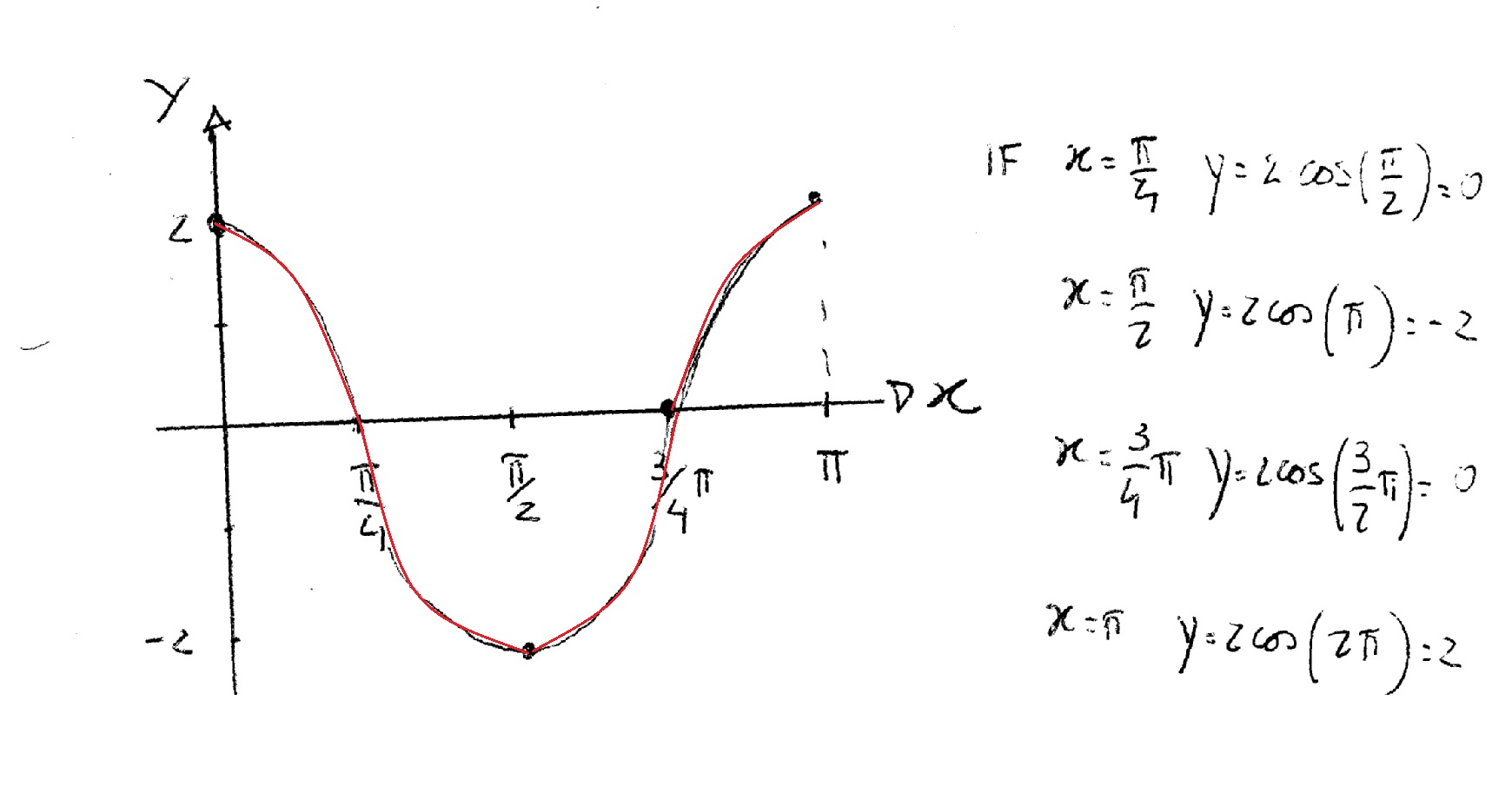



How Do You Graph Y 2cos2x Socratic



Pmt Physicsandmathstutor Com Download Maths A Level C3 Topic Qs Edexcel Set 1 C3 trigonometry trigonometric identities Pdf
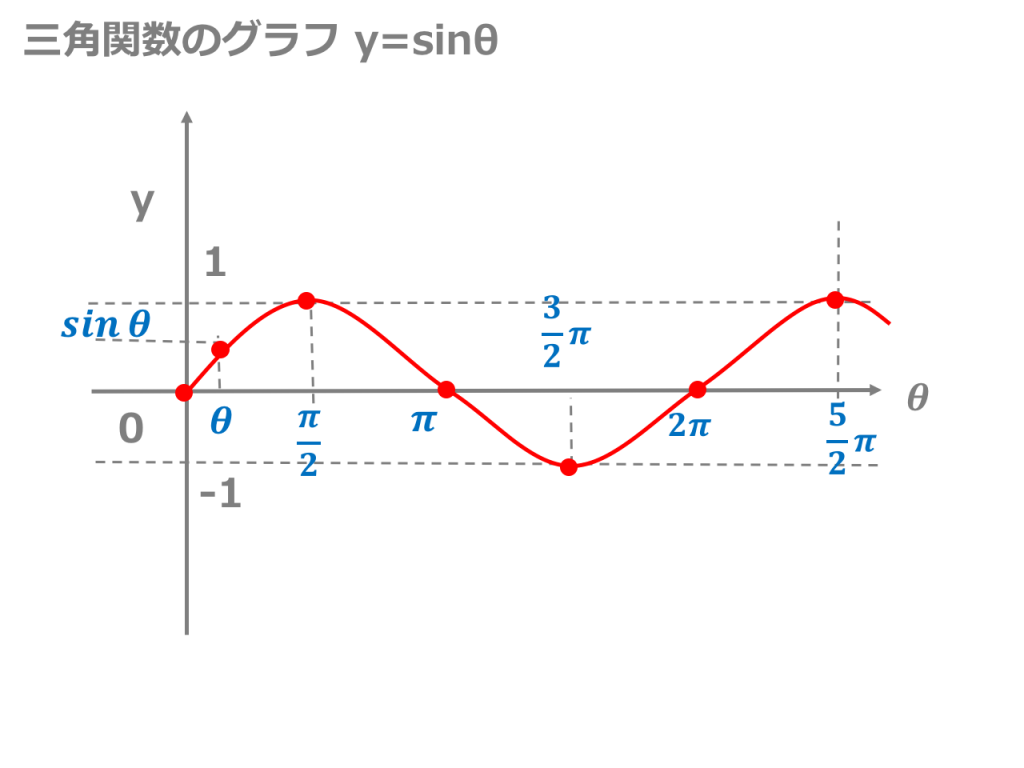



3分でわかる 三角関数のグラフの描き方 合格サプリ
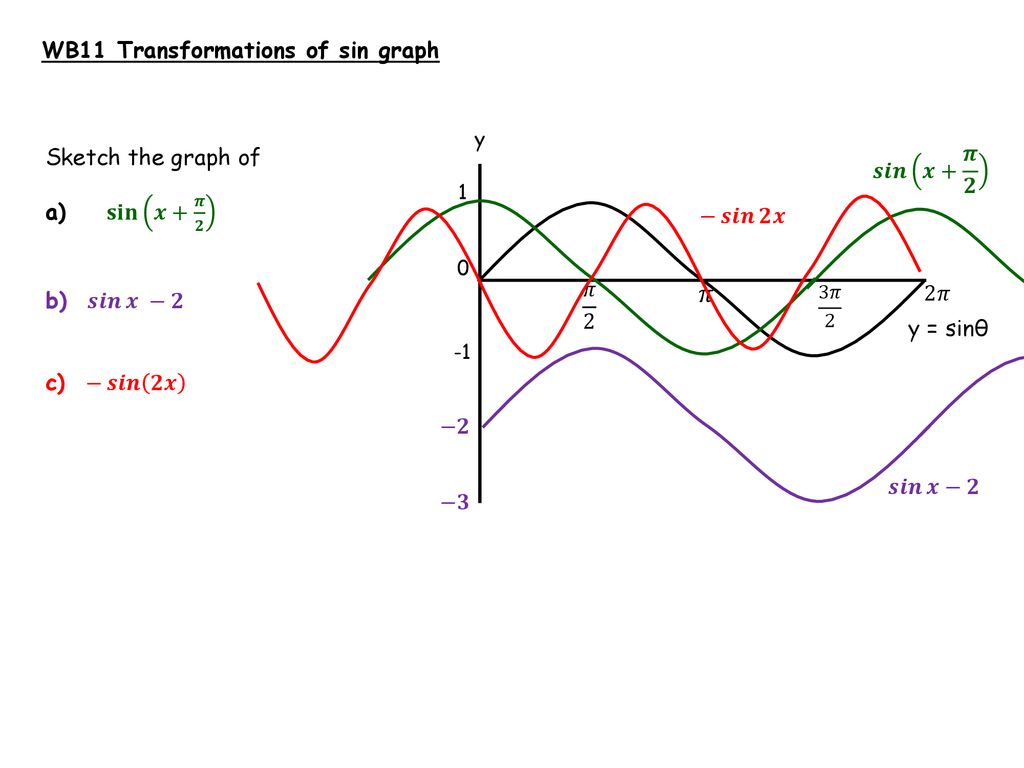



Trig Graphs And Equations Ppt Download




合格る一題 うかる一題 第1問 解答 解説 河合塾マナビス




From Now On This Is Strictly Second Year Work Ppt Download
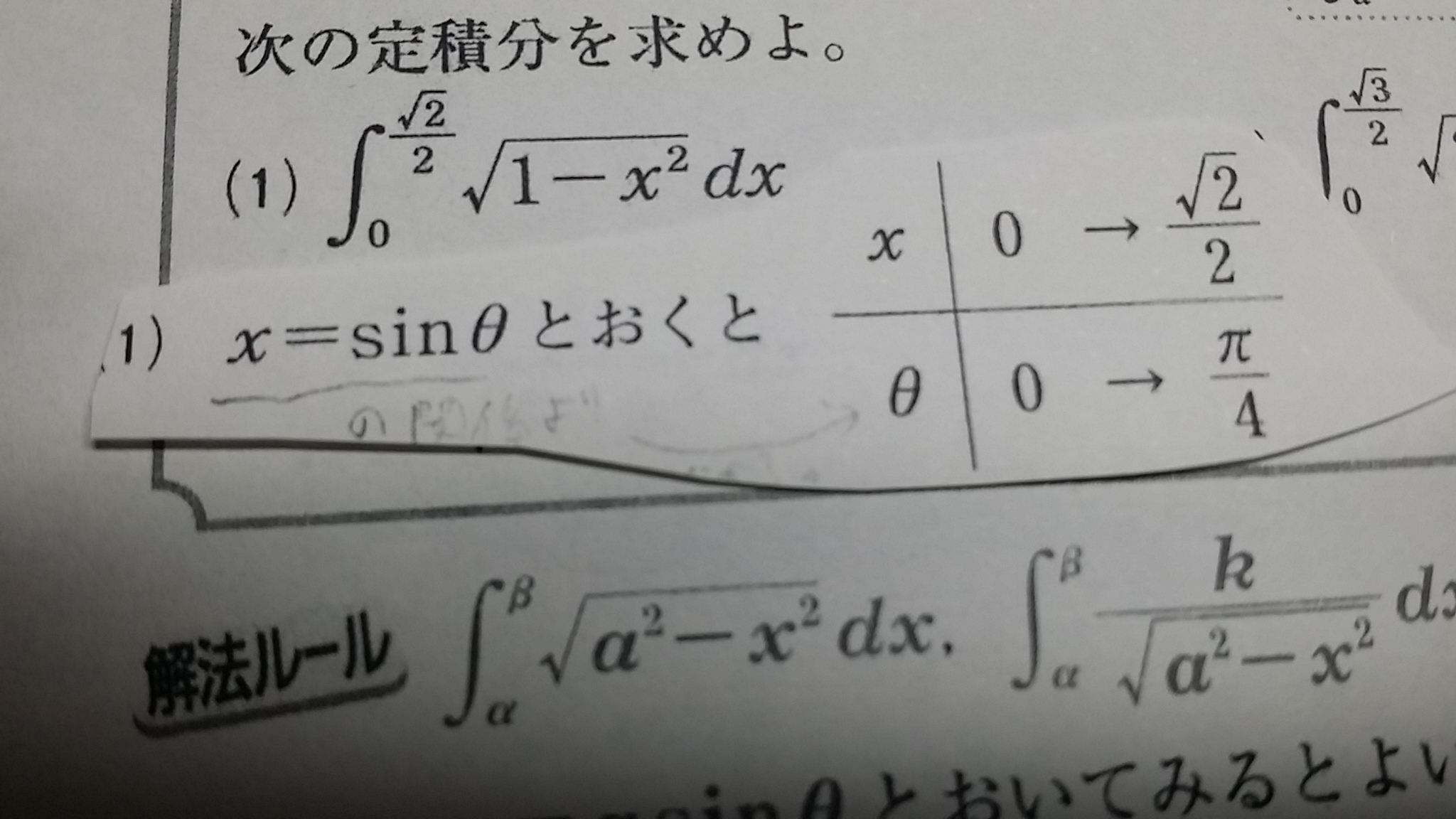



Sin8の8の範囲はなんで0 8 P 2と Okwave



Pplato Flap Math 1 6 Trigonometric Functions
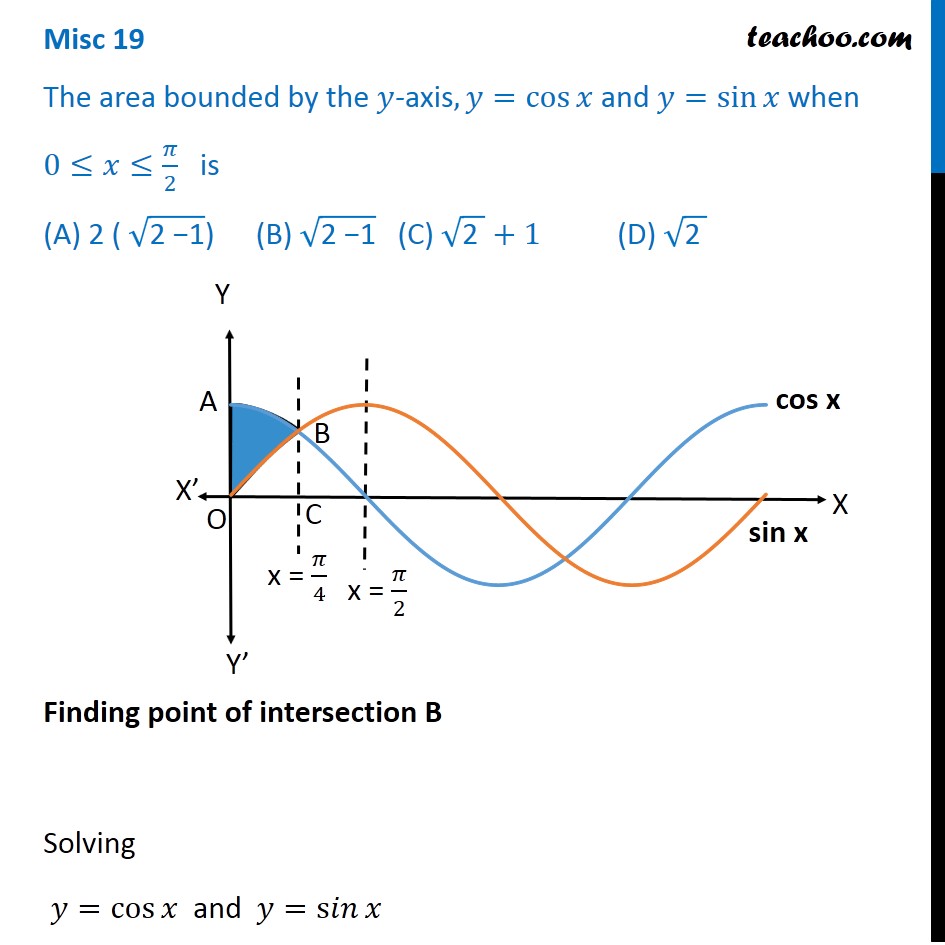



Misc 19 Area Bounded By Y Axis Y Cos X Y Sin X Miscellaneous



Vertical Tangent Line For R 1 Cos Theta Mathematics Stack Exchange



三角関数のグラフの書き方を徹底解説 周期や平行移動の問題も 受験辞典



Draw The Graph Of Y Sin 1 2xsqrt 1 X 2
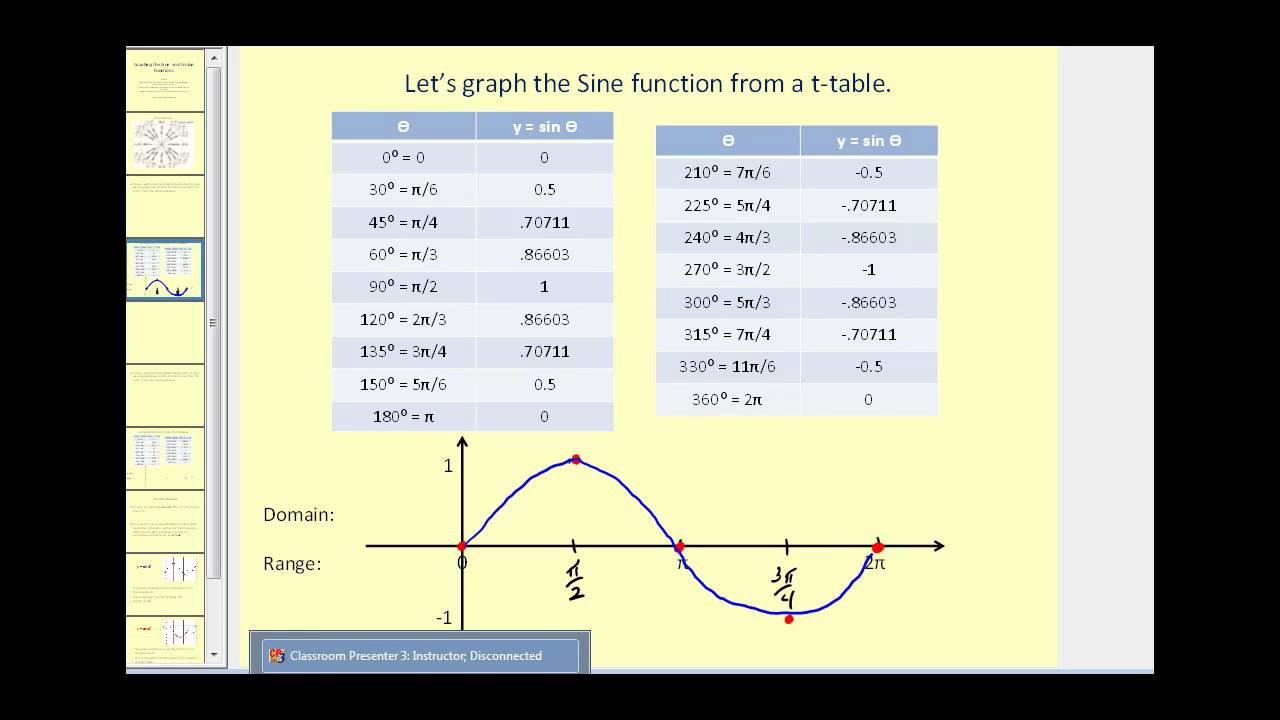



Sine Graph Solutions Examples Videos



Ppt 三角関数とそのグラフ Powerpoint Presentation Free Download Id 4758
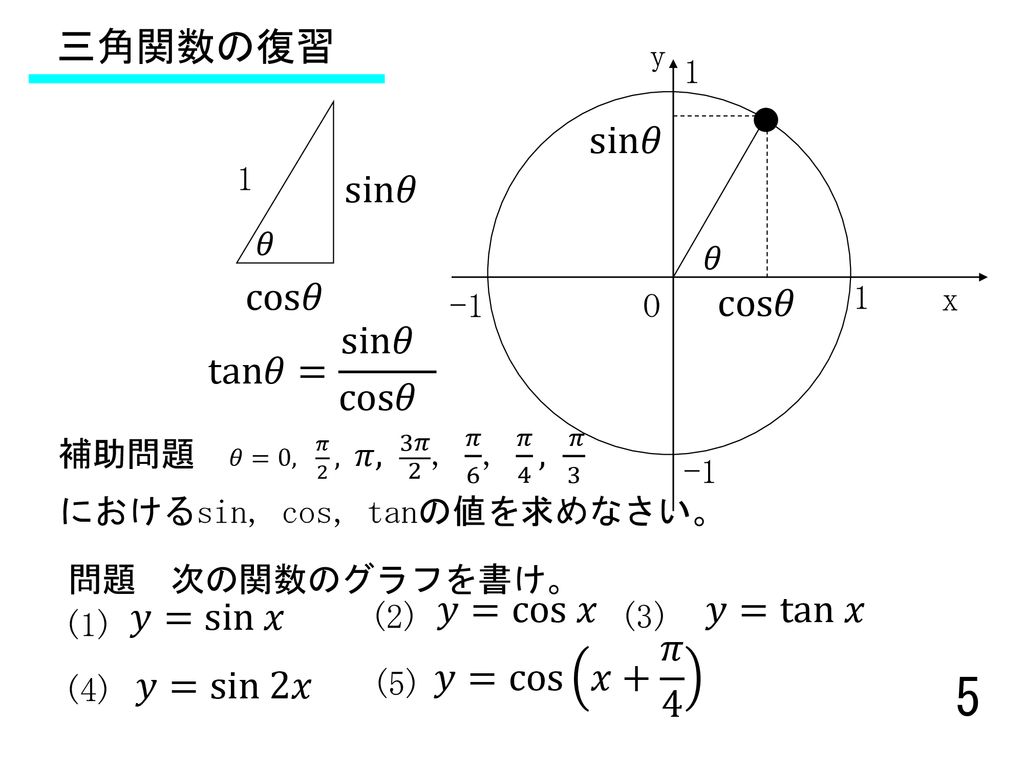



振動と波動の準備 三角関数の復習 定義と微分 三角関数は 物理でよく使います Ppt Download




Give The Smallest Value For A To Complete The Graph Mathematics Stack Exchange



Pmt Physicsandmathstutor Com Download Maths A Level C2 Topic Qs Edexcel Set 1 C2 trigonometry trigonometric equations Pdf




Y Sin28のグラフはy Sin8のグラフを2分の1倍に縮小させればいいことは分か Clear



交流瞬時値の三角関数表示式 音声付き電気技術解説講座 公益社団法人 日本電気技術者協会



物理と三角関数について2 三角関数のグラフ 高校物理 理解の手助け
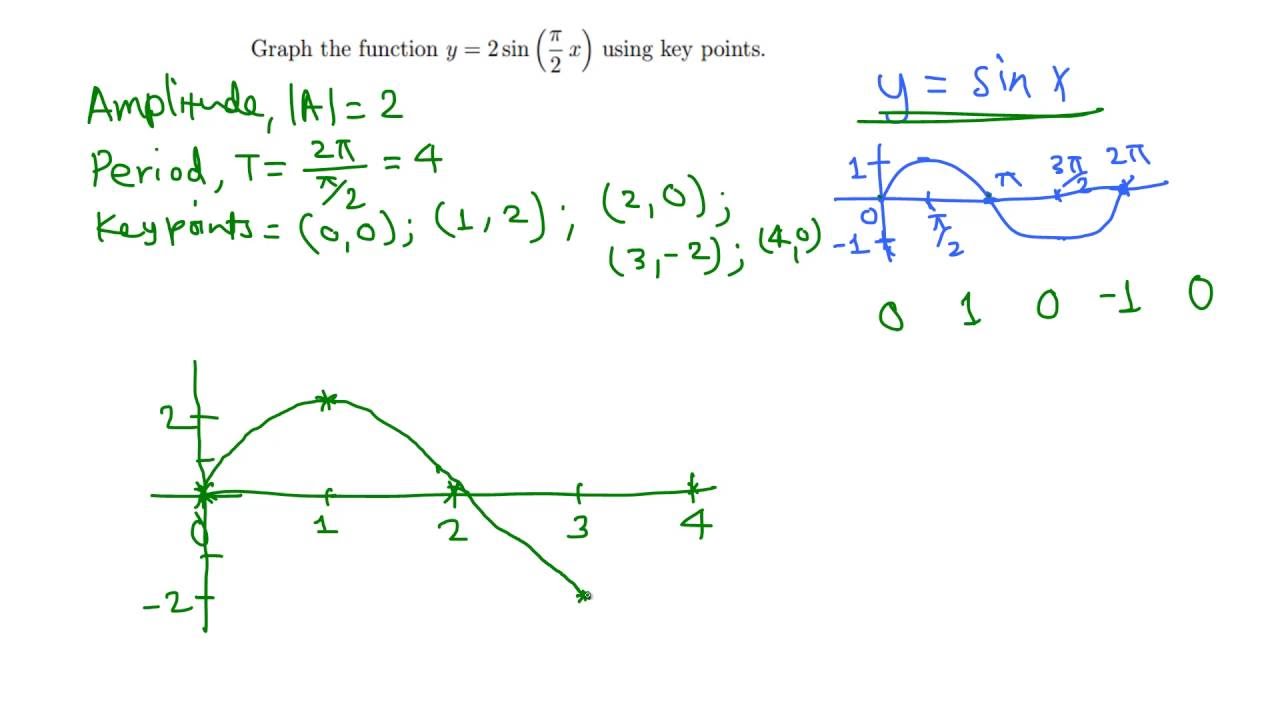



Graph Of Y 2 Sin Pi 2 X Using Key Points Youtube




7 のtanの式とグラフについてです Clear



Users Math Msu Edu Users Magyarp Math132 Notes 2 4 Trig Derivatives Pdf
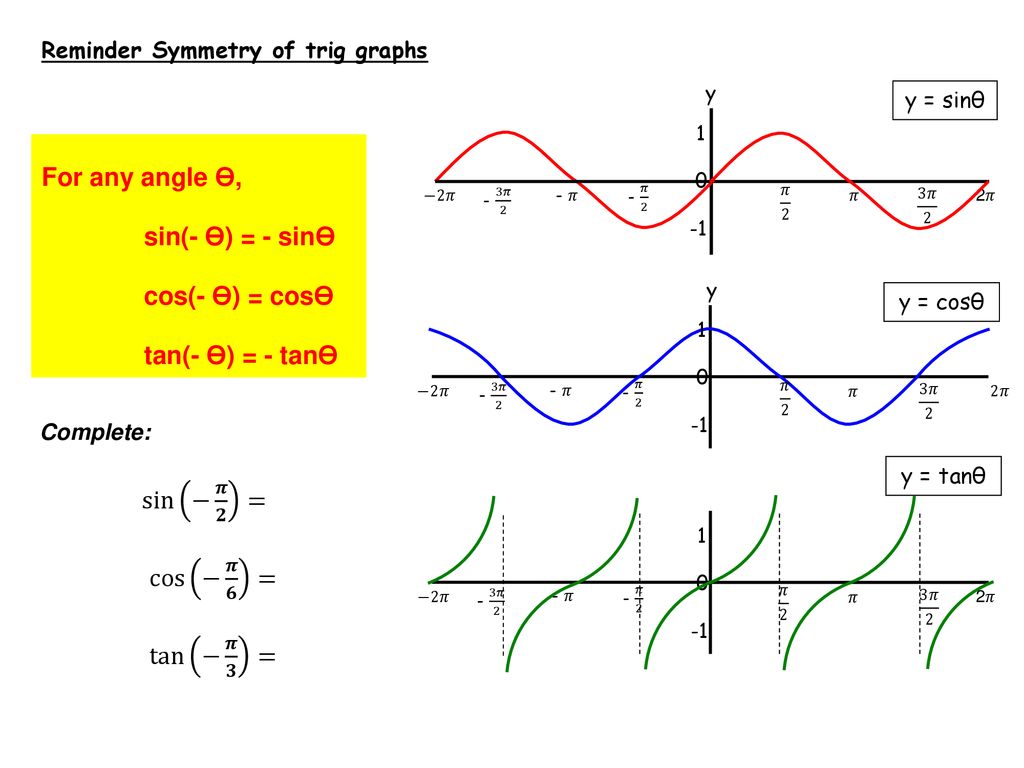



Trig Graphs And Equations Ppt Download



Math 222 Quiz 6 Take Home Solutions Problem 1 A 1 Point Use




この説明が全然理解できません Sinの方は 8 P 2 と言われているのに 何故si Clear
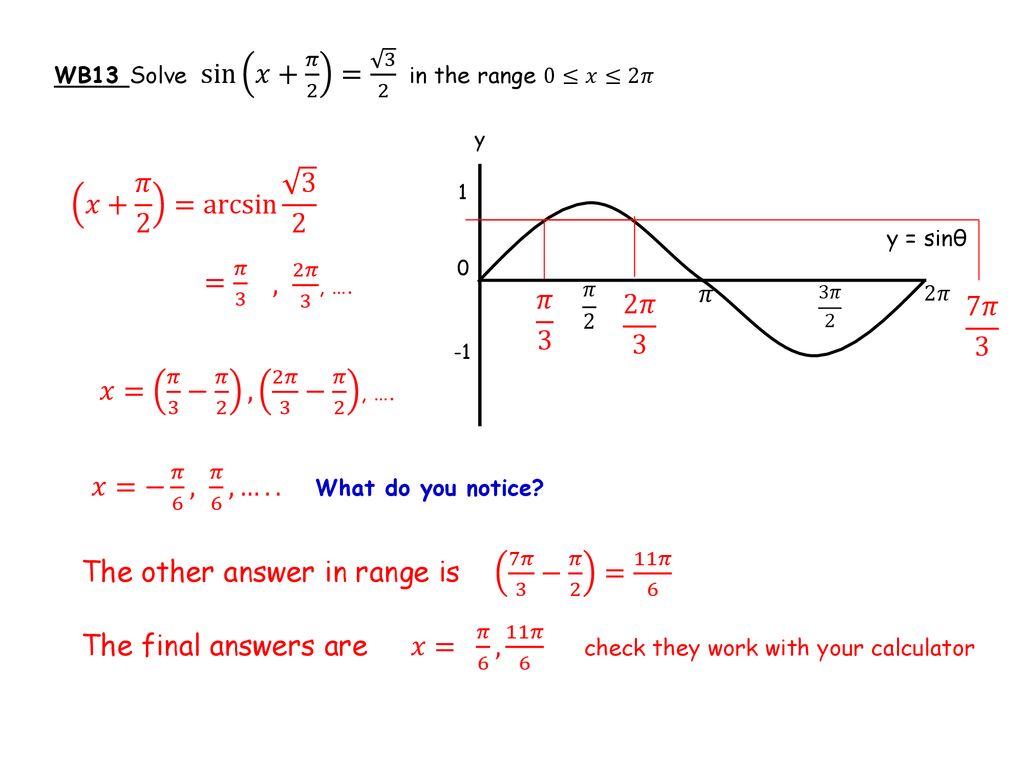



Trig Graphs And Equations Ppt Download




Math Polar Coordinates Ppt Download




Intersection Points Of Y Sin X And Y Cos X Video Khan Academy



Pplato Flap Math 1 6 Trigonometric Functions
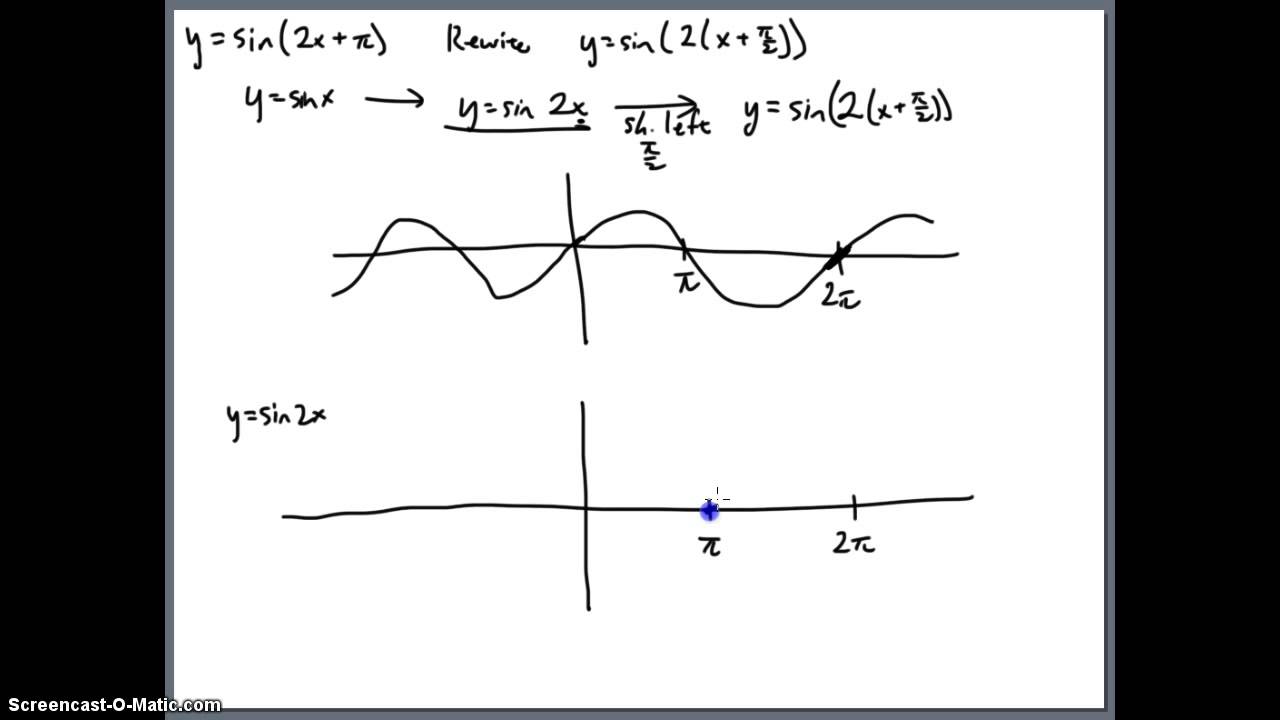



Y Sin 2x Pi Youtube
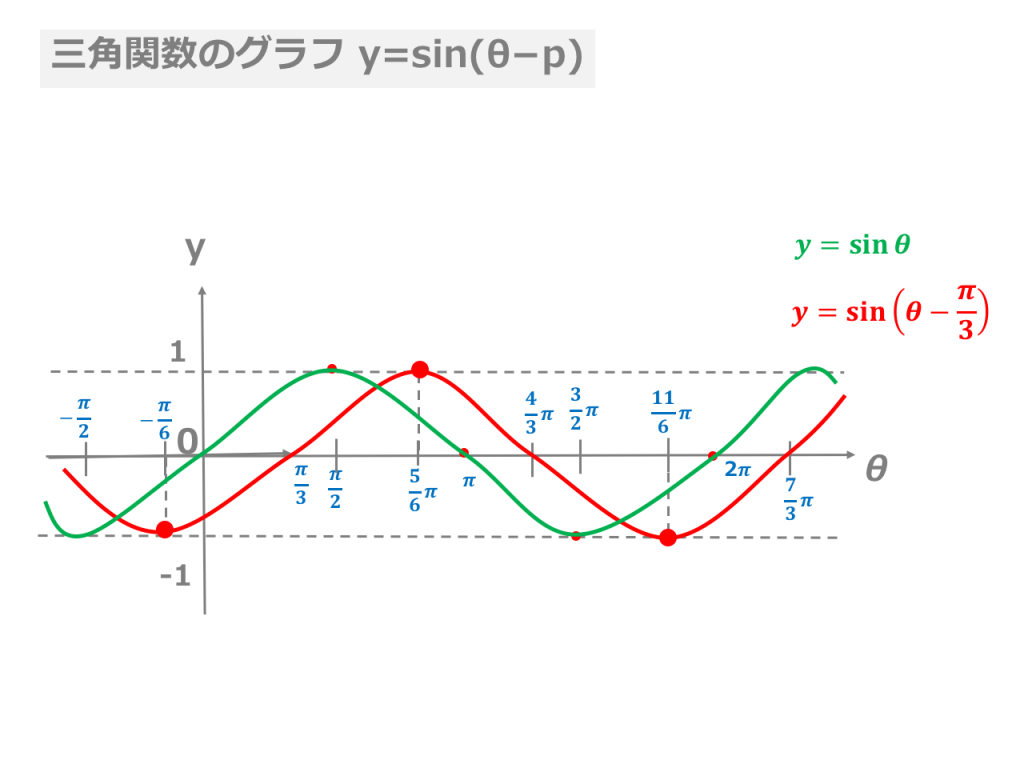



3分でわかる 三角関数のグラフの描き方 合格サプリ




Which Two Functions Will Have The Same Graph Y S Gauthmath



三角関数のグラフの書き方を徹底解説 周期や平行移動の問題も 受験辞典



3



三角関数のグラフの書き方を徹底解説 周期や平行移動の問題も 受験辞典



アステロイド曲線 星芒形 齊藤数学教室 算数オリンピックから大学数学入門




Sine Wikipedia
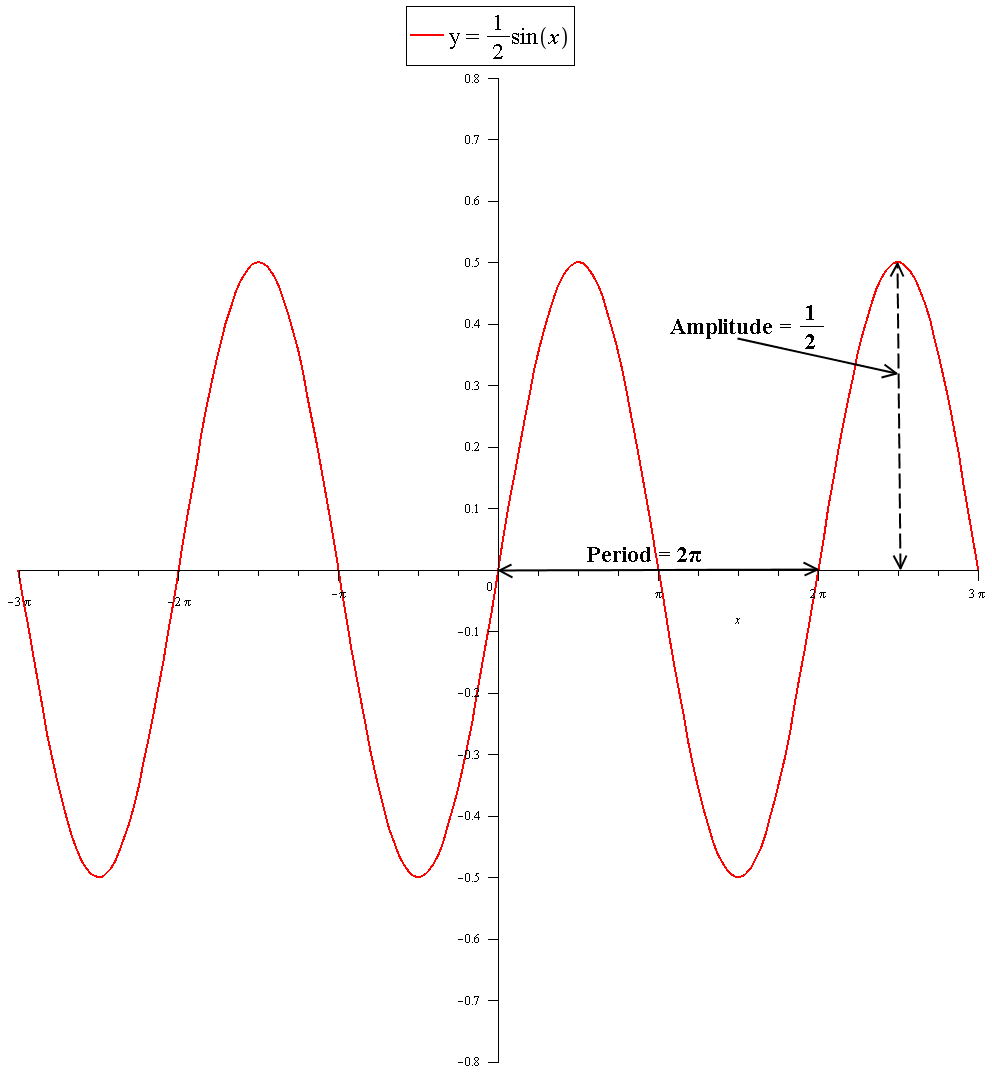



How Do You Find The Amplitude And Period Of Y 1 2sintheta Socratic




合格る一題 うかる一題 第1問 解答 解説 河合塾マナビス



48s96ub7b0z5f Net Sankakukansuu Graph 2



3分でわかる 三角関数のグラフの描き方 合格サプリ




三角関数のグラフの書き方とコツ Sin Cos Tan 周期 理系ラボ




The Curve R 2 Sin 2 8 Has A Slope At 8 P Gauthmath




Trigonometric Graph How To Draw Y 3 Sin 2x 1 Youtube



2




Trig Graphs And Equations Ppt Download



How Do You Find The Points Of Intersection Of Theta Pi 4 R 2 Socratic




Trigonometry Addition Formula For Rcos X A And R X A Ppt Download




三番の解説でt 1のときsin 8 P 4 1 2がわかりません なぜ1 2な Clear



3




Use The Graph To Find The Value Of Y Sin Theta For The Value Of Theta 1 4pi Radians A 0 7b Brainly Com



A Particle Moves Along The Curve Y Sin X Starting Chegg Com




Ppt 三角関数とそのグラフ Powerpoint Presentation Free Download Id 4758



3分でわかる 三角関数のグラフの描き方 合格サプリ




166 Y Sin48 167 Y Cos 8 6分のp Clear



Pplato Flap Math 3 2 Polar Representation Of Complex Numbers
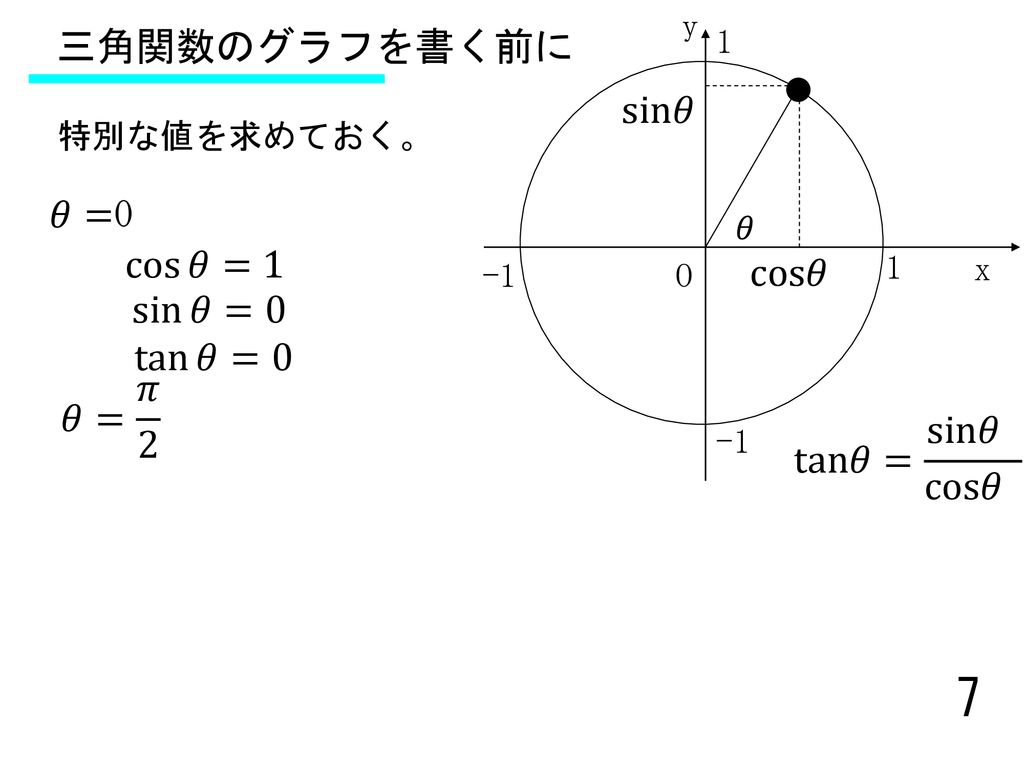



振動と波動の準備 三角関数の復習 定義と微分 三角関数は 物理でよく使います Ppt Download




なぜsin 8 3分のp 2分の1より 8 3分のp 4分の3p 4分の9p Clear




このグラフって8が変化するときのyの値 Sin8とかcos8 の値を求めて書いている Clear




166 Y Sin48 167 Y Cos 8 6分のp Clear




数学 Ii Yvmfz7d6a1tmvov تويتر
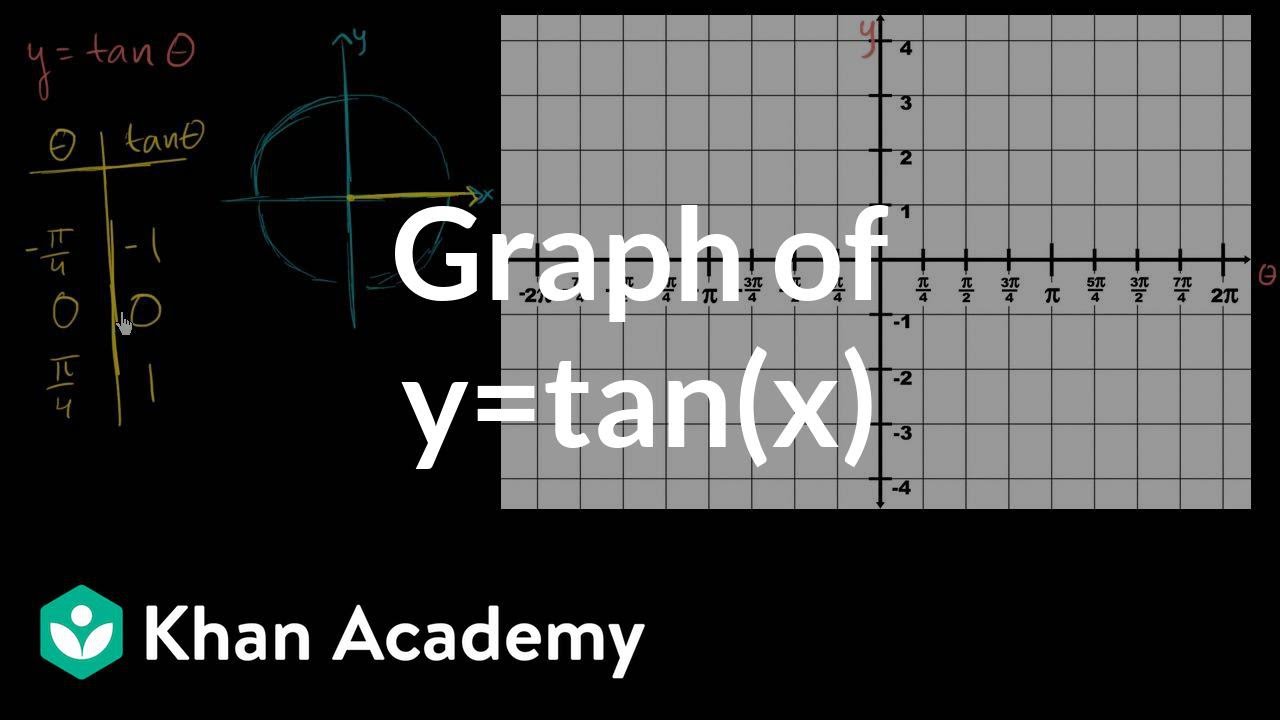



Graph Of Y Tan X Video Trigonometry Khan Academy
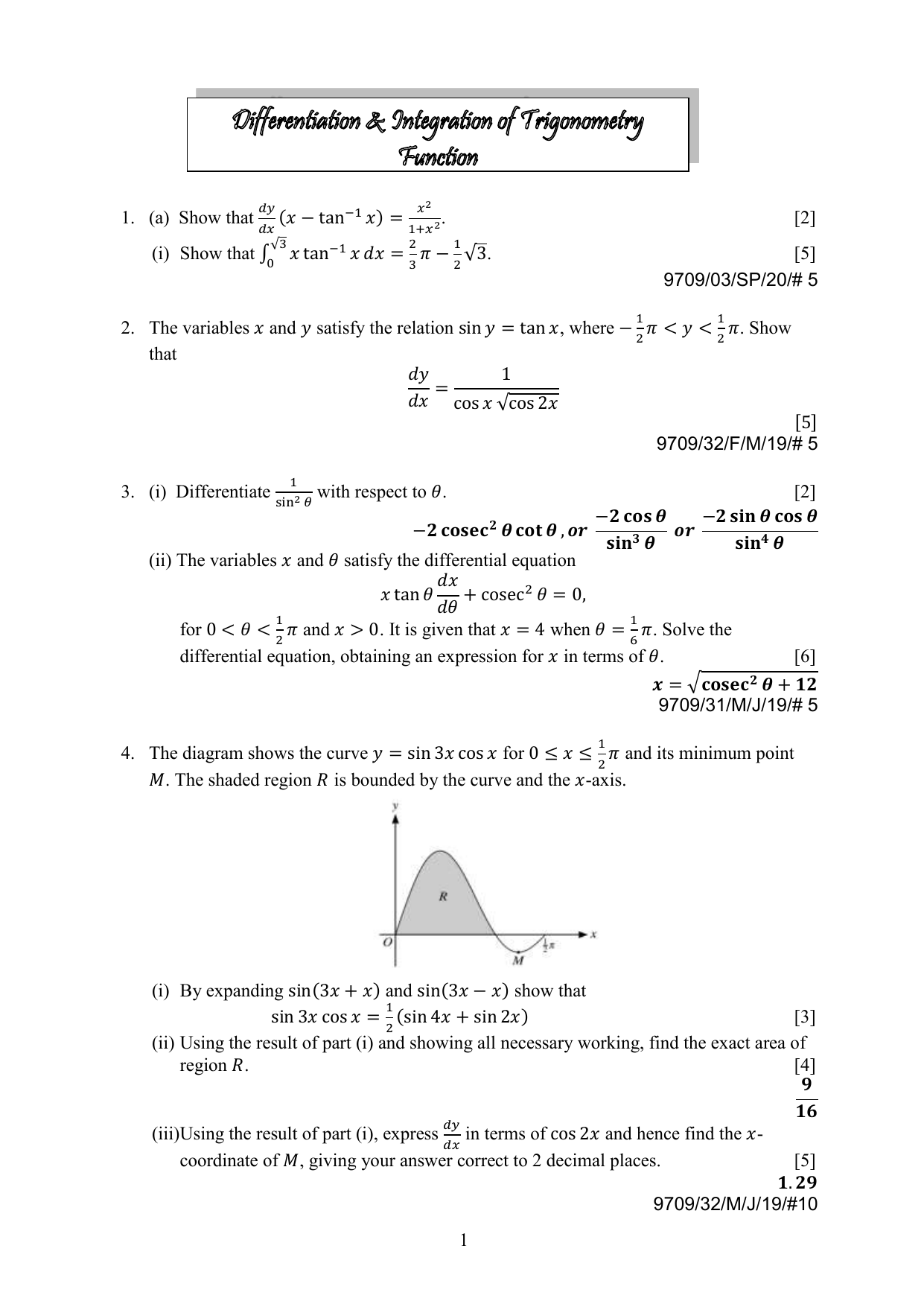



21 Differentiation And Integration Of Trigonometry Function 9709



1
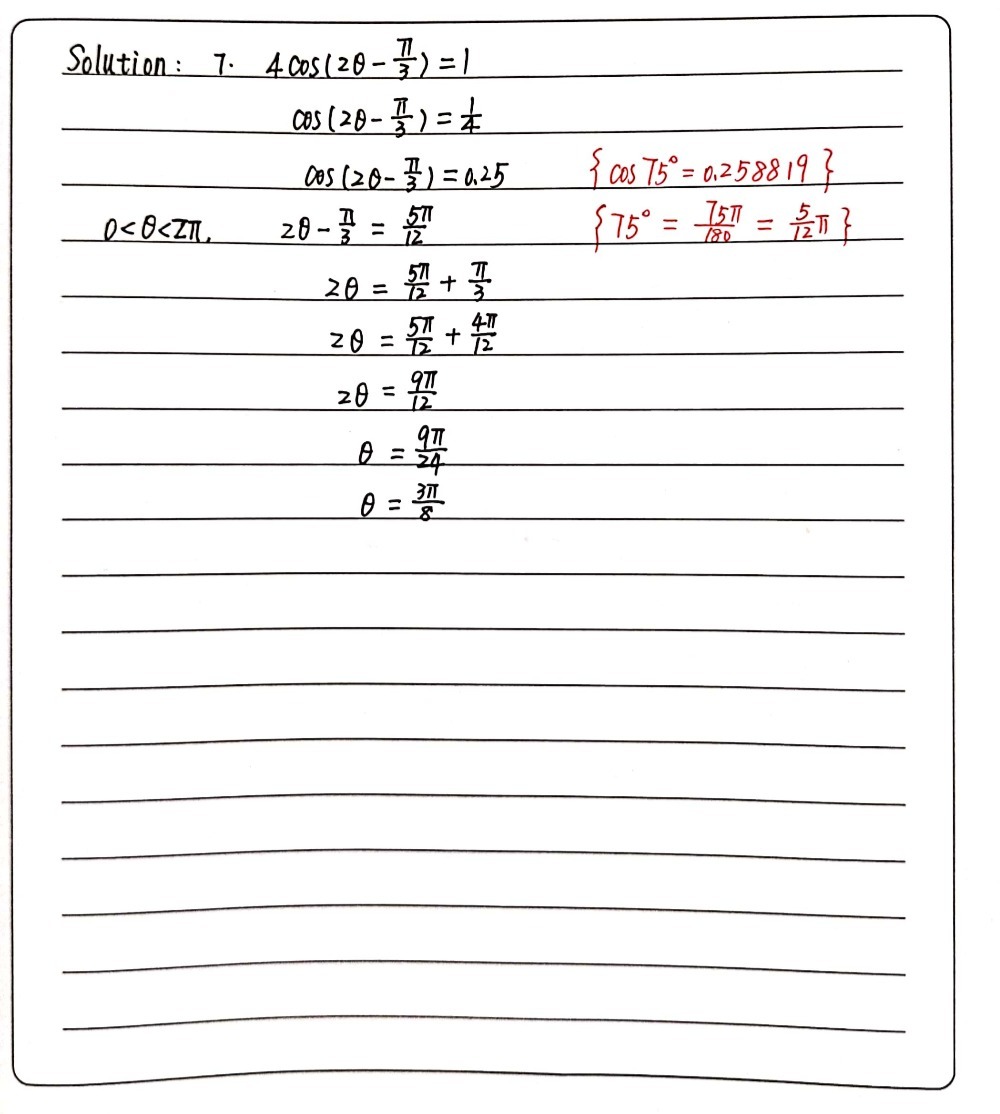



7 Solve 4cos 2 8 P 3 1 For 0 8 2 P Gauthmath
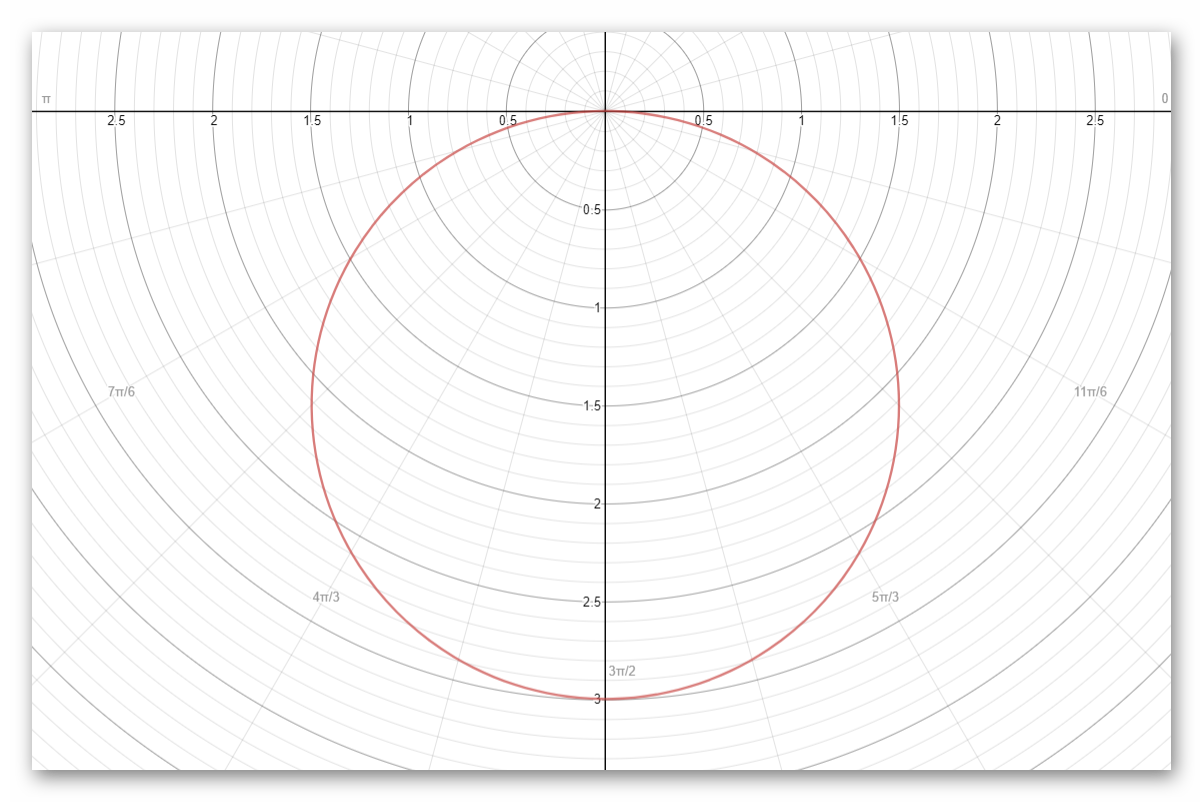



How Do You Graph The Polar Equation R 3sintheta Socratic




Transformation Of Cos X To Sin X Via Cos X Frac Pi 2 Sin X Mathematics Stack Exchange
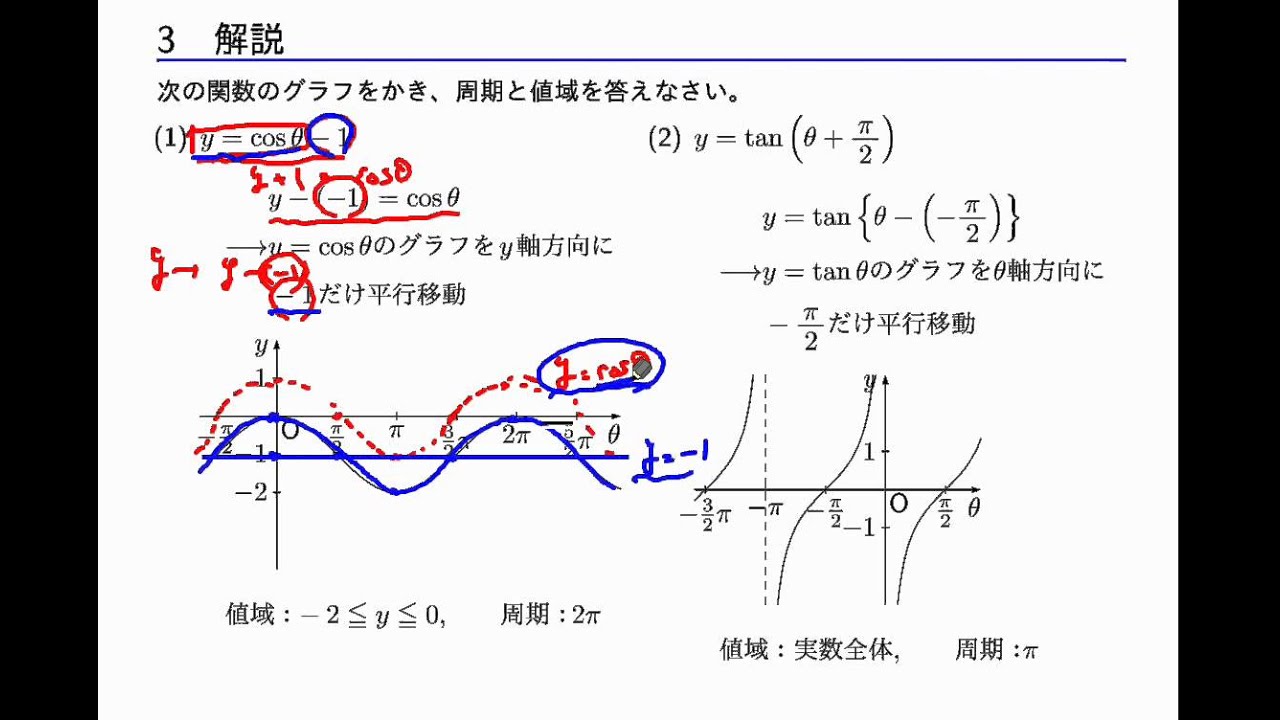



数学ii 三角関数 3 3 Y Sin 8 P Qのグラフ Youtube
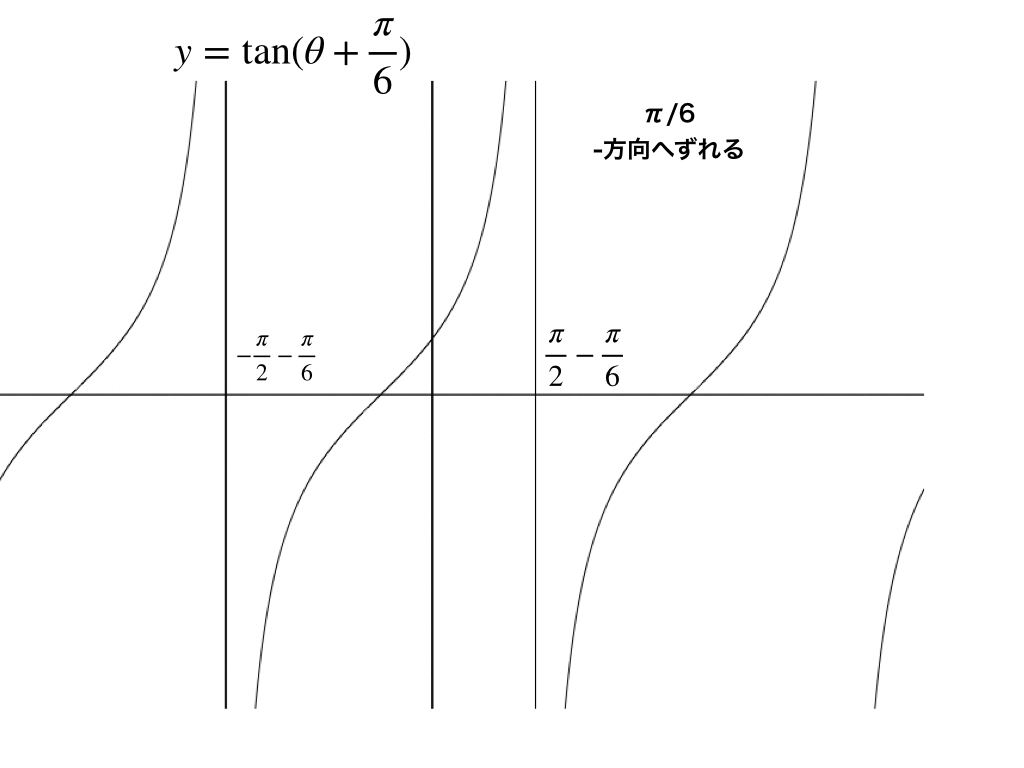



三角関数のグラフの描き方と式の平行移動 振幅 周期の読み取りを解説
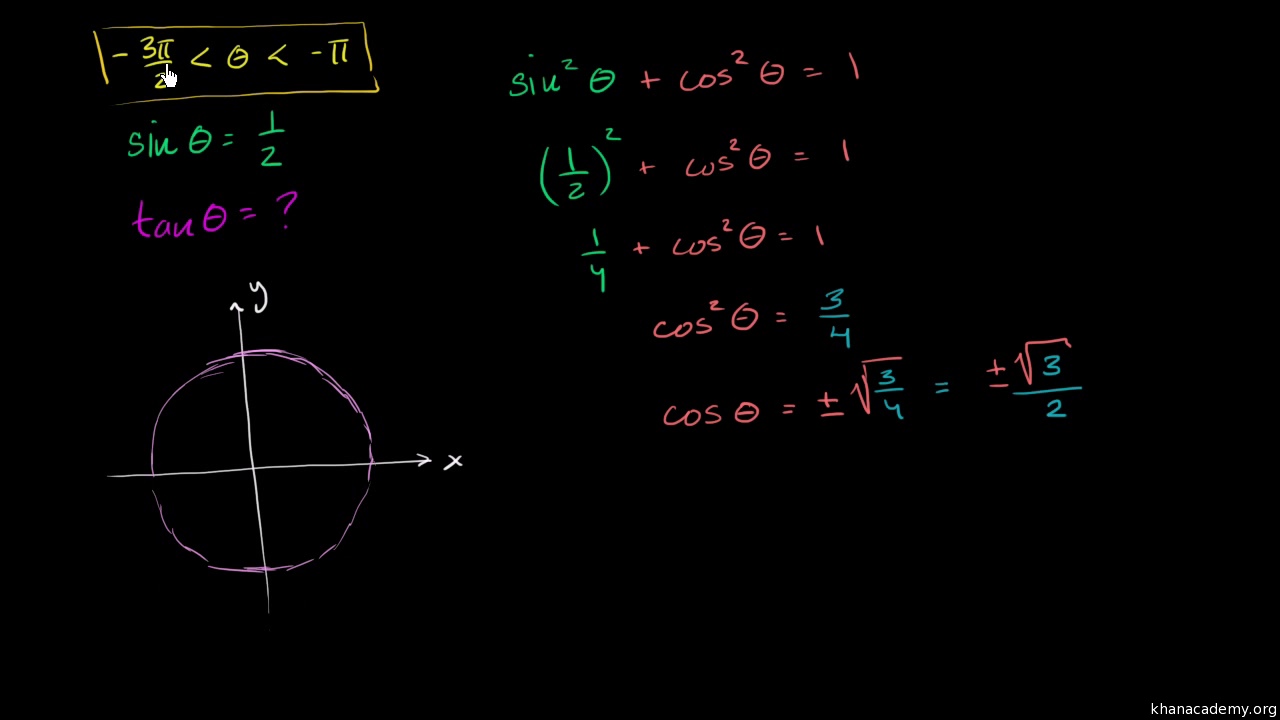



Using The Pythagorean Trig Identity Video Khan Academy



Bestmaths



0 件のコメント:
コメントを投稿